Question 2:
Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial:
(i) t2 – 3, 2t4 + 3t3 – 2t2 – 9t – 12
(ii) x2 + 3x + 1, 3x4 + 5x3 – 7x2 + 2x + 2
(iii) x3 – 3x + 1, x5 – 4x3 + x2 + 3x + 1
Answer:
(i) t2 – 3, 2t4 + 3t3 – 2t2 – 9t – 12
t2 – 3 = t2 + 0.t – 3
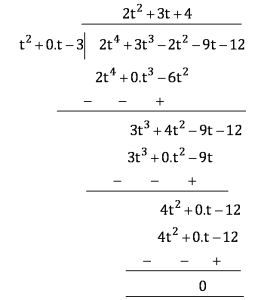
Since the remainder is 0,
Hence, t2 – 3 is a factor of 2t4 + 3t3 – 2t2 – 9t – 12.
(ii) x2 + 3x + 1, 3x4 + 5x3 – 7x2 + 2x + 2

Since the remainder is 0,
Hence, x2 + 3x + 1 is a factor of 3x4 + 5x3 – 7x2 + 2x + 2.
(iii) x3 – 3x + 1, x5 – 4x3 + x2 + 3x + 1
Since the remainder ≠ 0,
Hence, x3 – 3x + 1 is not a factor of x5 – 4x3 + x2 + 3x + 1.
Latest Govt Job & Exam Updates: