Question 7:

Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Answer:

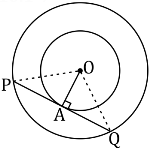
Let the two concentric circles be centered at point O. And let PQ be the chord of the larger circle which touches the smaller circle at point A. Therefore, PQ is tangent to the smaller circle.

OA ⊥ PQ (As OA is the radius of the circle)

Applying Pythagoras theorem in ΔOAP, we obtain

OA² + AP² = OP²

3² + AP² = 5²

9 + AP² = 25

AP² = 16

AP = 4

In ΔOPQ,

Since OA ⊥ PQ,

AP = AQ (Perpendicular from the center of the circle bisects the chord)

∴ PQ = 2AP = 2 × 4 = 8

Therefore, the length of the chord of the larger circle is 8 cm.

Latest Govt Job & Exam Updates:

View Full List ...