Question 1:
Solve the following pair of linear equations by the substitution method.

Answer:
(i) x + y = 14 (1)
x − y = 4 (2)
From (1), we obtain
x = 14 − y (3)
Substituting this value in equation (2), we obtain
(14 – y) – y = 4
14 – 2y = 4
10 = 2y
y = 5 (4)
Substituting this in equation (3), we obtain
x = 9
∴ x = 9, y = 5
(ii) s – t = 3 (1)

From (1), we obtain
s = t + 3
Substituting this value in equation (2), we obtain

Substituting in equation (3), we obtain
s = 9
∴ s = 9, t = 6
(iii)3x − y = 3 (1)
9x − 3y = 9 (2)
From (1), we obtain
y = 3x − 3 (3)
Substituting this value in equation (2), we obtain
9x – 3(3x – 3) = 9
9x – 9x + 9 = 9
9 = 9
This is always true.
Hence, the given pair of equations has infinite possible solutions and the relation between these variables can be given by
y = 3x − 3
Therefore, one of its possible solutions is x = 1, y = 0.
(iv) 0.2x + 0.3y = 1.3 (1)
0.4x + 0.5y = 2.3 (2)
From equation (1), we obtain
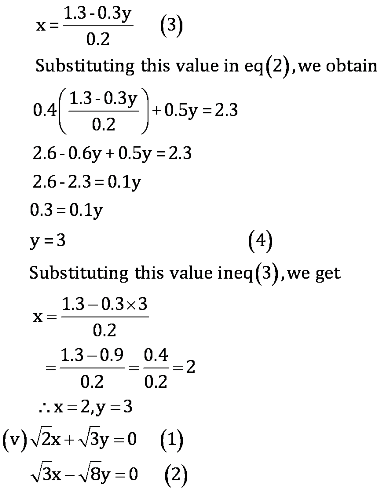

Substituting this value in equation (3), we obtain
x = 0
∴ x = 0, y = 0

Substituting this value in equation (3), we obtain

Latest Govt Job & Exam Updates: