Question 1:
Verify that the numbers given alongside of the cubic polynomials below are their zeroes. Also verify the relationship between the zeroes and the coefficients in each case:
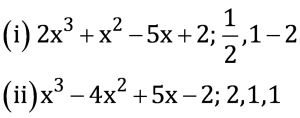
Answer:
(i) p(x) = 2x3 + x2 – 5x + 2.
Zeroes for this polynomials are 1/2, 1 –2.
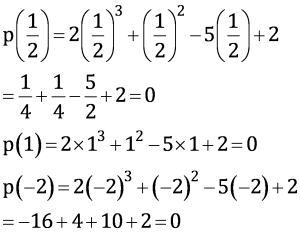
Therefore, 1/2, 1, and −2 are the zeroes of the given polynomial.
Comparing the given polynomial with ax3 + bx2 + cx + d, we obtain a = 2, b = 1, c = −5, d = 2

Therefore, the relationship between the zeroes and the coefficients is verified.
(ii) p(x) = x3 – 4x2 + 5x – 2
Zeroes for this polynomial are 2, 1, 1.
p(2) = 23 – 4(22) + 5(2) – 2
= 8 – 16 + 10 – 2 = 0
p(1) = 13 – 4(1)2 + 5(1) – 2
= 1 – 4 + 5 – 2 = 0
Therefore, 2, 1, 1 are the zeroes of the given polynomial.
Comparing the given polynomial with ax3 + bx2 + cx + d, we obtain a = 1, b = −4, c = 5, d = −2.
Verification of the relationship between zeroes and coefficient of the given polynomial
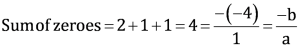
Multiplication of zeroes taking two at a time = (2)(1) + (1)(1) + (2)(1) =2 + 1 + 2 = 5 = (5)/1 = c/a
Multiplication of zeroes = 2 × 1 × 1 = 2 ![]()
Hence, the relationship between the zeroes and the coefficients is verified.
Latest Govt Job & Exam Updates: