Question 2:

Find a quadratic polynomial each with the given numbers as the sum and product of its zeroes respectively.

(i)   1/4, –1           (ii) √2, 1/3          (iii) 0, √5

(iv)  1,1               (v) –1/4, ¼           (vi) 4, 1

Answer:

(i)   1/4, –1

Let the polynomial be ax² + bx + c, and its zeroes be  α and β.

 

If a = 4, then b = -1, c = -4

Therefore, the quadratic polynomial is 4x² − x − 4.

(ii) √2, 1/3

Let the polynomial be ax² + bx + c, and its zeroes be  α and β.

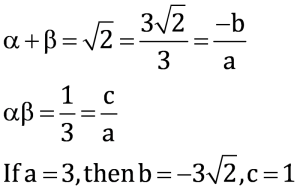
Therefore, the quadratic polynomial is 3x² − 3√2x + 1.

 (iii) 0, √5

Let the polynomial be ax² + bx + c, and its zeroes be  α and β.

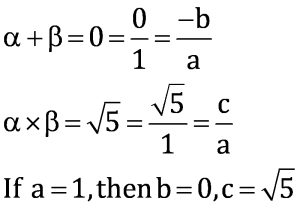
Therefore, the quadratic polynomial is x² + √5.

(iv)  1,1

Let the polynomial be ax² + bx + c, and its zeroes be  α and β.

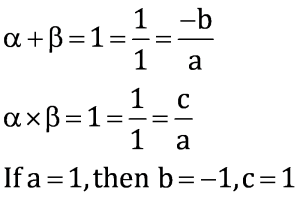
Therefore, the quadratic polynomial is x² – x + 1.

(v) –1/4, ¼

Let the polynomial be ax² + bx + c, and its zeroes be  α and β.

Therefore, the quadratic polynomial is 4x² + x + 1.

(vi) 4 , 1

Let the polynomial be ax² + bx + c.

Therefore, the quadratic polynomial is x² – 4x + 1.

Latest Govt Job & Exam Updates:

View Full List ...