JEE-Advanced-2020-Question-Paper-1 Code-E
PHYSICS
Section 1 (Maximum Marks:18)
• This Section contains Four (06) Questions.
• Each question has FOUR options. ONLY ONE of these four options is the correct answer.
• For each question, Choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen;
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered)
Negative Marks : −1 In all other cases.
1. A football of radius R is kept on a hole of radius r (r < R) made on a plank kept horizontally. One end of the plank is now lifted so that it gets tilted making an angle θ from the horizontal as shown in the figure below. The maximum value of θ so that the football does not start rolling down the plank satisfies (the figure is schematic and not drawn to scale)
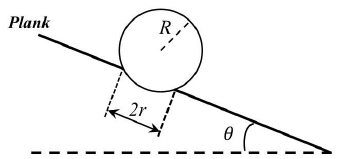
(A) sin θ = r/R
(B) tan θ = r/R
(C) sin θ = r/2R
(D) cos θ = r/2R
2. A light disc made of aluminium (a nonmagnetic material) is kept horizontally and is free to rotate about its axis as shown in the figure. A strong magnet is held vertically at a point above the disc away from its axis. On revolving the magnet about the axis of the disc, the disc will (the figure is schematic and not drawn to scale);
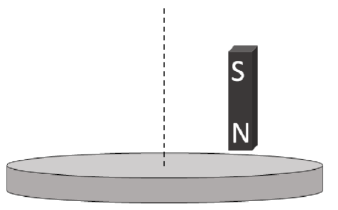
(A) rotate in the direction opposite to the direction of magnet’s motion
(B) rotate in the same direction as the direction of magnet’s motion
(C) not rotate and its temperature will remain unchanged
(D) not rotate but its temperature will slowly rise
3. A small roller of diameter 20 cm has an axle of diameter 10 cm (see figure below on the left). It is on a horizontal floor and a meter scale is positioned horizontally on its axle with one edge of the scale on top of the axle (see figure on the right). The scale is now pushed slowly on the axle so that it moves without slipping on the axle, and the roller starts rolling without slipping. After the roller has moved 50 cm, the position of the scale will look like (figures are schematic and not drawn to scale);
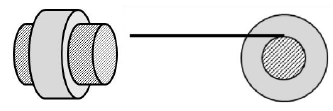
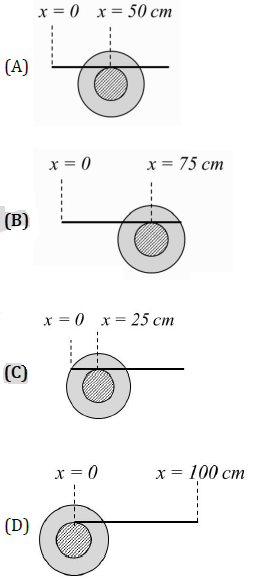
4. A circular coil of radius R and N turns has negligible resistance. As shown in the schematic figure, its two ends are connected to two wires and it is hanging by those wires with its plane being vertical. The wires are connected to a capacitor with charge Q through a switch. The coil is in a horizontal uniform magnetic field Bo parallel to the plane of the coil. When the switch is closed, the capacitor gets discharged through the coil in a very short time. By the time the capacitor is discharged fully, magnitude of the angular momentum gained by the coil will be (assume that the discharge time is so short that the coil has hardly rotated during this time)
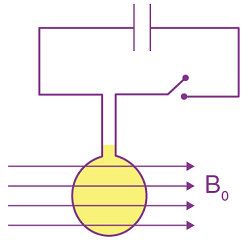
(A) 
(B) πNQB0R2
(C) 2πNQB0R2
(D) 4πNQB0R2
5. A parallel beam of light strikes a piece of transparent glass having cross section as shown in the figure below. Correct shape of the emergent wave front will be (figures are schematic and not drawn to scale)
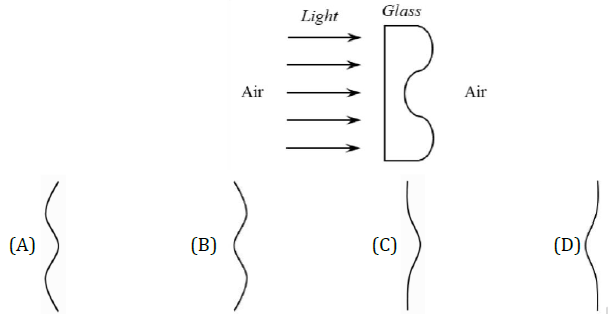
6. An open-ended U-tube of uniform cross-sectional area contains water (density 103kg m−3). Initially, the water level stands at 0.29 m from the bottom in each arm. Kerosene oil (a water-immiscible liquid) of density 800 kg m−3 is added to the left arm until its length is 0.1 m, as shown in the schematic figure below. The ratio (h1/ h2) of the heights of the liquid in the two arms is
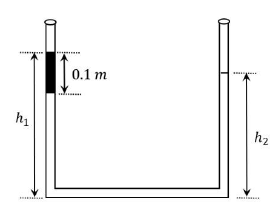
(A) 15/14
(B) 35/33
(C) 7/6
(D) 5/4
SECTION 2 (Maximum Marks: 24)
• This section contains SIX (06) questions.
• Each question has FOUR options. ONE OR MORE THAN ONE of these four option(s) is (are) the correct answer(s).
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 If only (all) the correct option(s) is (are) chosen;
Partial Marks : +3 If all four options is correct but ONLY three options are chosen;
Partial Marks : +2 If there or more options are correct but ONLY two options are chosen; both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If none of the options is chosen (i.e. the questions is unanswered);
Negative Marks: −2 In all other cases.
7. A particle of mass m moves in circular orbits with potential energy V(r) = Fr, where F is a positive constant and r is its distance from the origin. Its energies are calculated using the Bohr model. If the radius of the particle’s orbit is denoted by R and its speed and energy are denoted by v and E, respectively, then for the nth orbit (here h is the Planck’s constant)
(A) R ∝ 11/3 and v ∝ n2/3
(B) R ∝ n2/3 and v ∝ n1/3
(C)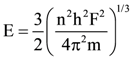 
(D)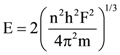 
8. The filament of a light bulb has a surface area 64 mm2. The filament can be considered as a black body at temperature 2500 K emitting radiation like a point source when viewed from far. At night the light bulb is observed from a distance of 100 m. Assume the pupil of the eyes of the observer to be circular with radius 3 mm. Then
(Take Stefan-Boltzmann constant = 5.67 × 10−8 Wm−2K−4,
Wien’s displacement constant = 2.90 × 10−3m-K,
Planck’s constant = 6.63 × 10−34Js,
speed of light in vacuum = 3.00 ×108 ms−1)
(A) power radiated by the filament is in the range 642 W to 645 W
(B) radiated power entering into one eye of the observer is in the range 3.15 × 10−8 W to 3.25 × 10−8 W
(C) the wavelength corresponding to the maximum intensity of light is 1160 nm
(D) taking the average wavelength of emitted radiation to be 1740 nm, the total number of photons entering per second into one eye of the observer is in the range 2.75 × 1011 to 2.85 × 1011
9. Sometimes it is convenient to construct a system of units so that all quantities can be expressed in terms of only one physical quantity. In one such system, dimensions of different quantities are given in terms of a quantity X as follows: [position] = [Xα]; [speed] = [Xβ]; [acceleration] =[Xp]; [linear momentum] = [Xq]; [force] = [Xr]. Then
(A) α + 𝑝 = 2β
(B) p + q − r = β
(C) − q + r = α
(D) p + q + r = β
10. A uniform electric field, 
is applied in a region. A charged particle of mass m carrying positive charge q is projected in this region with an initial speed of 2√10 × 106 ms−1. his particle is aimed to hit a target T, which is 5 m away from its entry point into the field as shown schematically in the figure. Take q/m = 1010CKg−1. Then
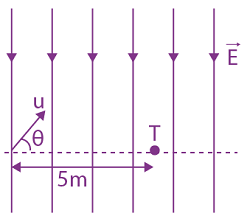
(A) the particle will hit T if projected at an angle 45° from the horizontal
(B) the particle will hit T if projected either at an angle 30° or 60° from the horizontal
(C) time taken by the particle to hit T could be 
(D) time taken by the particle to hit T is 
11. Shown in the figure is a semicircular metallic strip that has thickness t and resistivity ρ. Its inner radius is R1 and outer radius is R2. If a voltage V0 is applied between its two ends, a current I flows in it. In addition, it is observed that a transverse voltage ∆V develops between its inner and outer surfaces due to purely kinetic effects of moving electrons (ignore any role of the magnetic field due to the current). Then (figure is schematic and not drawn to scale)
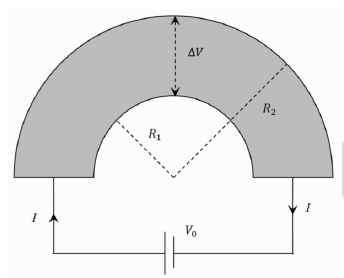
(A)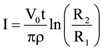 
(B) the outer surface is at a higher voltage than the inner surface
(C) the outer surface is at a lower voltage than the inner surface
(D) ∆VαI2
12. As shown schematically in the figure, two vessels contain water solutions (at temperature T) of potassium permanganate (KMnO4) of different concentrations 𝑛1 and n2(n1 > n2) molecules per unit volume with ∆𝑛 = (n1 – n2) ≪ n1. When they are connected by a tube of small length l and cross-sectional area S, KMnO4 starts to diffuse from the left to the right vessel through the tube. Consider the collection of molecules to behave as dilute ideal gases and the difference in their partial pressure in the two vessels causing the diffusion. The speed v of the molecules is limited by the viscous force −βv on each molecule, where β is a constant. Neglecting all terms of the order (∆n)2, which of the following is/are correct? (kB is the Boltzmann constant)
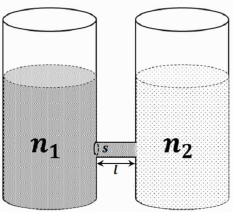
(A) the force causing the molecules to move across the tube is ∆nkBTS
(B) force balance implies n1βvl = ∆nkBT
(C) total number of molecules going across the tube per sec is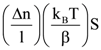 
(D) rate of molecules getting transferred through the tube does not change with time
SECTION-3 (Maximum Marks: 24)
• This section contains SIX (06) questions. The answer to each question is a NUMERICAL VALUE.
•For Each question, enter the correct numerical value of the answer using the mouse and the on-screen virtual numerical keypad in the place designated to enter the answer. If the numerical value has more than two decimal places. truncate/round-off the value to TWO decimal places.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +4 If ONLY the correct numerical value is entered;
Zero Marks : 0 In all other cases.
13. Put a uniform meter scale horizontally on your extended index fingers with the left one at 0.00 cm and the right one at 90.00 cm. When you attempt to move both the fingers slowly towards the centre, initially only the left finger slips with respect to the scale and the right finger does not. After some distance, the left finger stops and the right one start slipping. Then the right finger stops at a distance xR from the centre (50.00 cm) of the scale and the left one starts slipping again. This happens because of the difference in the frictional forces on the two fingers. If the coefficients of static and dynamic friction between the fingers and the scale are 0.40 and 0.32, respectively, the value of xR (in cm) is ______.
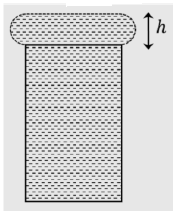
14. When water is filled carefully in a glass, one can fill it to a height h above the rim of the glass due to the surface tension of water. To calculate h just before water starts flowing, model the shape of the water above the rim as a disc of thickness h having semicircular edges, as shown schematically in the figure. When the pressure of water at the bottom of this disc exceeds what can be withstood due to the surface tension, the water surface breaks near the rim and water starts flowing from there. If the density of water, its surface tension and the acceleration due to gravity are 103kg m−3, 0.07 Nm−1 and 10 ms−2, respectively, the value of h (in mm) is _________.
15. One end of a spring of negligible unstretched length and spring constant k is fixed at the origin (0, 0). A point particle of mass m carrying a positive charge q is attached at its other end. The entire system is kept on a smooth horizontal surface. When a point dipole 𝑝 pointing towards the charge q is fixed at the origin, the spring gets stretched to a length l and attains a new equilibrium position (see figure below). If the point mass is now displaced slightly by ∆l ≪ 𝑙from its equilibrium position and released, it is found to oscillate at frequency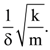 
The value of δ is ______.
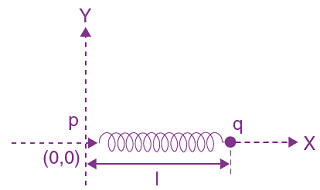
16. Consider one mole of helium gas enclosed in a container at initial pressure P1 and volume V1. It expands isothermally to volume 4V1. After this, the gas expands adiabatically and its volume becomes 32V1. The work done by the gas during isothermal and adiabatic expansion processes are Wiso and Wadia, respectively. If the ratio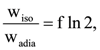 
then f is ________.
17. A stationary tuning fork is in resonance with an air column in a pipe. If the tuning fork is moved with a speed of 2 ms−1 in front of the open end of the pipe and parallel to it, the length of the pipe should be changed for the resonance to occur with the moving tuning fork. If the speed of sound in air is 320 ms−1, the smallest value of the percentage change required in the length of the pipe is ____________.
18. A circular disc of radius R carries surface charge density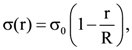 
where 𝜎0 is a constant and r is the distance from the center of the disc. Electric flux through a large spherical surface that encloses the charged disc completely is ϕ0. Electric flux through another spherical surface of radius R/4 and concentric with the disc is ϕ. Then the ratio 
is _________.
CHEMISTRY
Section 1 (Maximum Marks:18)
• This Section contains Four (06) Questions.
• Each question has FOUR options. ONLY ONE of these four options is the correct answer.
• For each question, Choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen;
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered)
Negative Marks : −1 In all other cases.
1. If the distribution of molecular speeds of gas is as per the figure shown below, then the ratio of the most probable, the average, and the root mean square speeds, respectively, is
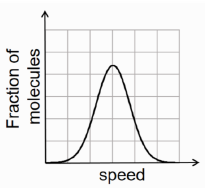
(A) 1 : 1 : 1
(B) 1 : 1 : 1.224
(C) 1 : 1.128 : 1.224
(D) 1 : 1.128 : 1
2. Which of the following liberates O2 upon hydrolysis?
(A) Pb3O2
(B) KO2
(C) Na2O2
(D) Li2O2
3. A colourless aqueous solution contains nitrates of two metals, X and Y. When it was added to an aqueous solution of NaCl, a white precipitate was formed. This precipitate was found to be partially soluble in hot water to give a residue P and a solution Q. The residue P was soluble in aq. NH3 and also in excess sodium thiosulfate. The hot solution Q gave a yellow precipitate with KI. The metals X and Y, respectively, are
(A) Ag and Pb
(B) Ag and Cd
(C) Cd and Pb
(D) Cd and Zn
4. Newman projections P, Q, R and S are shown below:
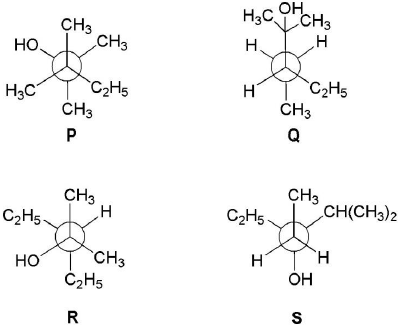
Which one of the following options represents identical molecules?
(A) P and Q
(B) Q and S
(C) Q and R
(D) R and S
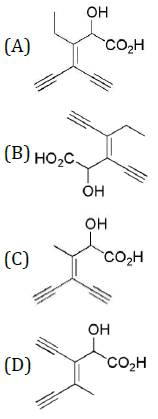
5. Which one of the following structures has the IUPAC name 3-ethynyl-2-hydroxy-4-methylhex-3-en-5-ynoic acid?
6. The Fischer projection of D-erythrose is shown below.
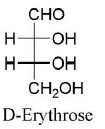
D-Erythrose and its isomers are listed as P, Q, R, and S in Column-I. Choose the correct relationship of P, Q, R, and S with D-erythrose from Column II.
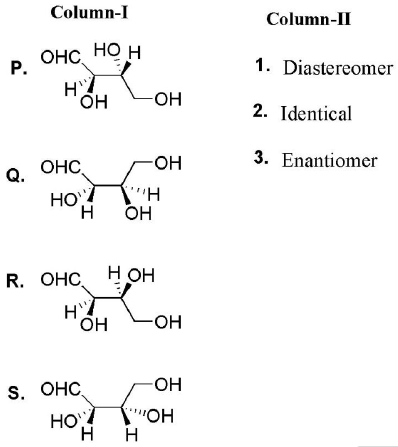
(A) P→2, Q→3, R→2, S→2
(B) P→3, Q→1, R→1, S→2
(C) P→2, Q→1, R→1, S→3
(D) P→2, Q→3, R→3, S→1
SECTION 2 (Maximum Marks: 24)
• This section contains SIX (06) questions.
• Each question has FOUR options. ONE OR MORE THAN ONE of these four option(s) is (are) the correct answer(s).
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 If only (all) the correct option(s) is (are) chosen;
Partial Marks : +3 If all four options is correct but ONLY three options are chosen;
Partial Marks : +2 If there or more options are correct but ONLY two options are chosen; both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If none of the options is chosen (i.e. the questions is unanswered);
Negative Marks: −2 In all other cases.
7. In thermodynamics, the P − V work done is given by w = −∫dVPext
For a system undergoing a particular process, the work done is,
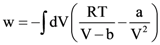
This equation is applicable to a
(A) System that satisfies the van der Waals equation of state.
(B) Process that is reversible and isothermal.
(C) Process that is reversible and adiabatic.
(D) Process that is irreversible and at constant pressure.
8. With respect to the compounds I-V, choose the correct statement(s).
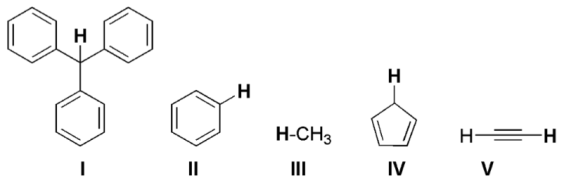
(A) The acidity of compound I is due to delocalization in the conjugate base.
(B) The conjugate base of compound IV is aromatic.
(C) Compound II becomes more acidic, when it has a -NO2 substituent.
(D) The acidity of compounds follows the order I >IV>V>II>III.
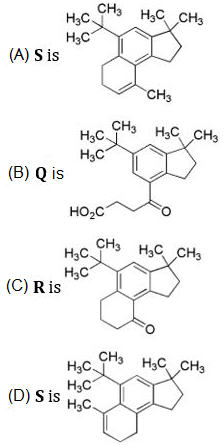
9. In the reaction scheme shown below, Q, R, and S are the major products.
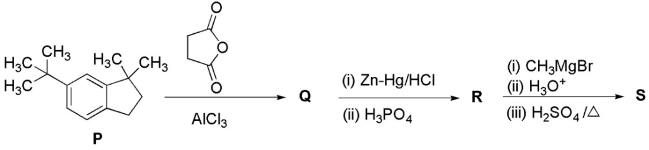
The correct structure of
10. Choose the correct statement(s) among the following:
(A) [FeCl4]− has tetrahedral geometry.
(B) [Co(en)(NH3)2Cl2]+has 2 geometrical isomers.
(C) [FeCl4]− has higher spin-only magnetic moment than [Co(en)(NH3)2Cl2]+.
(D) The cobalt ion in [Co(en)(NH3)2Cl2]+ has sp3d2 hybridization.
11. With respect to hypochlorite, chlorate and perchlorate ions, choose the correct statement(s).
(A) The hypochlorite ion is the strongest conjugate base.
(B) The molecular shape of only chlorate ion is influenced by the lone pair of electrons of Cl.
(C) The hypochlorite and chlorate ions disproportionate to give rise to an identical set of ions.
(D) The hypochlorite ion oxidizes the sulfite ion.
12. The cubic unit cell structure of a compound containing cation M and anion X is shown below. When compared to the anion, the cation has a smaller ionic radius. Choose the correct statement(s).
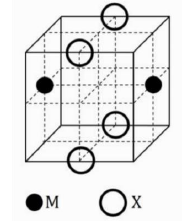
(A) The empirical formula of the compound is MX.
(B) The cation M and anion X have different coordination geometries.
(C) The ratio of M-X bond length to the cubic unit cell edge length is 0.866.
(D) The ratio of the ionic radii of cation M to anion X is 0.414.
SECTION-3 (Maximum Marks: 24)
• This section contains SIX (06) questions. The answer to each question is a NUMERICAL VALUE.
• For Each question, enter the correct numerical value of the answer using the mouse and the on-screen virtual numerical keypad in the place designated to enter the answer. If the numerical value has more than two decimal places. truncate/round-off the value to TWO decimal places.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +4 If ONLY the correct numerical value is entered;
Zero Marks : 0 In all other cases.
13. 5.00 mL of 0.10 M oxalic acid solution taken in a conical flask is titrated against NaOH from a burette using phenolphthalein indicator. The volume of NaOH required for the appearance of permanent faint pink color is tabulated below for five experiments. What is the concentration, in molarity, of the NaOH solution?
| Exp. No. | Vol. of NaOH
(mL) |
| 1 | 12.5 |
| 2 | 10.5 |
| 3 | 9.0 |
| 4 | 9.0 |
| 5 | 9.0 |
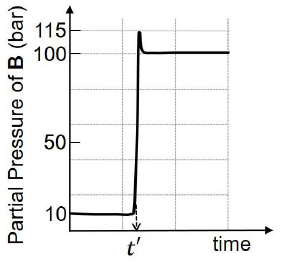
14. Consider the reaction A ⇌ B at 1000 K. At time 𝑡’, the temperature of the system was increased to 2000 K and the system was allowed to reach equilibrium. Throughout this experiment, the partial pressure of A was maintained at 1 bar. Given below is the plot of the partial pressure of B with time.
What is the ratio of the standard Gibbs energy of the reaction at 1000 K to that at 2000 K?
15. Consider a 70% efficient hydrogen-oxygen fuel cell working under standard conditions at 1 bar and 298 K. Its cell reaction is
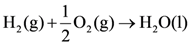
The work derived from the cell on the consumption of 1.0 × 10−3 mol of H2(g) is used to compress 1.00 mol of a monoatomic ideal gas in a thermally insulated container. What is the change in the temperature (in K) of the ideal gas?
The standard reduction potentials for the two half-cells are given below.
O2(g) + 4H+(aq) + 4e− → 2H2O (l), E° = 1.23 V,
2H+(aq) + 2e− → H2(g), E° = 0.00 V
Use F = 96500 C mol−1, R = 8.314 J mol−1 K−1.
16. Aluminum reacts with sulfuric acid to form aluminum sulfate and hydrogen. What is the volume of hydrogen gas in liters (L) produced at 300 K and 1.0 atm pressure, when 5.4 g of aluminum and 50.0 mL of 5.0 M sulfuric acid are combined for the reaction?
(Use molar mass of aluminum as 27.0 g mol−1, R = 0.082 atm L mol−1 K−1)
17. 
is known to undergo radioactive decay to form 
by emitting alpha and beta particles. A rock initially contained 68 × 10−6 g of 
If the number of alpha particles that it would emit during its radioactive decay of 
in three half-lives is Z × 1018, then what is the value of Z?
18. In the following reaction, compound Q is obtained from compound P via an ionic intermediate.
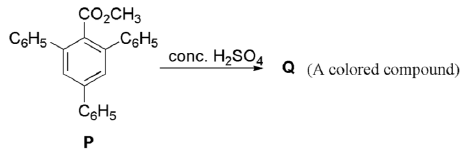
What is the degree of unsaturation of Q?
MATHEMATICS
Section 1 (Maximum Marks:18)
• This Section contains Four (06) Questions.
• Each question has FOUR options. ONLY ONE of these four options is the correct answer.
• For each question, Choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen;
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered)
Negative Marks : −1 In all other cases.
1. Suppose a, b denote the distinct real roots of the quadratic polynomial x2 + 20x − 2020 and suppose c, d denote the distinct complex roots of the quadratic polynomial x2 – 20x + 2020. Then the value of ac(a – c) + ad(a – d) + bc(b – c) + bd(b – d) is
(A) 0
(B) 8000
(C) 8080
(D) 16000
2. If the function f: R ⟶ R is defined by (x) = |x| (x – sinx), then which of the following statements is TRUE?
(A) f is one-one, but NOT onto
(B) f is onto, but NOT one-one
(C) f is BOTH one-one and onto
(D) f is NEITHER one-one NOR onto
3. Let the functions: R ⟶ R and g : R ⟶ R be defined by
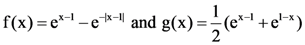
Then, the area of the region in the first quadrant bounded by the curves y = (x), y = g(x) and x = 0 is
(A) 
(B) 
(C) 
(D) 
4. Let a, b and λ be positive real numbers. Suppose P is an end point of the latus rectum of the parabola y2 = 4λx, and suppose the ellipse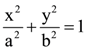 
passes through the point𝑃. If the tangents to the parabola and the ellipse at the point P are perpendicular to each other, then the eccentricity of the ellipse is
(A) 1/√2
(B) 1/2
(C) 1/3
(D) 2/5
5. Let C1 and C2 be two biased coins such that the probabilities of getting head in a single toss are 2/3 and 1/3, respectively. Suppose α is the number of heads that appear when C1 is tossed twice, independently, and suppose α is the number of heads that appear when C2 is tossed twice, independently. Then the probability that the roots of the quadratic polynomial x2 − αx + β are real and equal, is
(A) 40/81
(B) 20/81
(C) 1/2
(D) 1/4
6. Consider all rectangles lying in the region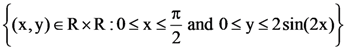
and having one side on the x-axis. The area of the rectangle which has the maximum perimeter among all such rectangles, is
(A) 3π/2
(B) π
(C) π/2√3
(D) π√3/2
SECTION 2 (Maximum Marks: 24)
• This section contains SIX (06) questions.
• Each question has FOUR options. ONE OR MORE THAN ONE of these four option(s) is (are) the correct answer(s).
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 If only (all) the correct option(s) is (are) chosen;
Partial Marks : +3 If all four options is correct but ONLY three options are chosen;
Partial Marks : +2 If there or more options are correct but ONLY two options are chosen; both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If none of the options is chosen (i.e. the questions is unanswered);
Negative Marks: −2 In all other cases.
7. Let the function f : R → R be defined by (x) = x3 − x2 + (x − 1) sin 𝑥 and let g : R → R be an arbitrary function. Let fg : R → R be the product function defined by (fg)(𝑥) = f(x)g(x). Then which of the following statements is/are TRUE?
(A) If g is continuous at x = 1, then fg is differentiable at x = 1
(B) If fg is differentiable at x = 1, then g is continuous at x = 1
(C) If g is differentiable at x = 1, then fg is differentiable at x = 1
(D) If fg is differentiable at x = 1, then g is differentiable at x = 1
8. Let M be a 3 × 3 invertible matrix with real entries and let I denote the 3 × 3 identity matrix. If M−1 = adj (adj M), then which of the following statements is/are ALWAYS TRUE?
(A) M = I
(B) det M = 1
(C) M2 = I
(D) (adj M)2 = I
9. Let S be the set of all complex numbers z satisfying |z2 + z + 1| = 1. Then which of the following statements is/are TRUE?
(A) 
(B) |z| ≤ 2 for all z ∈ S
(C) 
(D) The set S has exactly four elements
10. Let x, y and z be positive real numbers. Suppose x, y and z are the lengths of the sides of a triangle opposite to its angles X, Y and Z, respectively. If 
then which of the following statements is/are TRUE?
(A) 2Y = X + Z
(B) Y = X + Z
(C) 
(D) x2 + z2 – y2 = xz
11. Let L1 and L2 be the following straight line 
.
Suppose the straight line 
Lies in the plane containing L1 and L2, and passes through the point of intersection of L1 and L2. If the line L bisects the acute angle between the lines L1 and L2, then which of the following statements is/are TRUE?
(A) α − γ
(B) l + m = 2
(C) α – γ = 1
(D) l + m = 0
12. Which of the following inequalities is/are TRUE?
(A) 
(B) 
(C) 
(D) 
SECTION-3 (Maximum Marks: 24)
• This section contains SIX (06) questions. The answer to each question is a NUMERICAL VALUE.
• For Each question, enter the correct numerical value of the answer using the mouse and the on-screen virtual numerical keypad in the place designated to enter the answer. If the numerical value has more than two decimal places. truncate/round-off the value to TWO decimal places.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +4 If ONLY the correct numerical value is entered;
Zero Marks : 0 In all other cases.
13. Let m be the minimum possible value of 
where y1, y2, y3 are real numbers for which y1 + y2 + y3 = 9. Let M be the maximum possible value of (log3x1 + log3x2 + log3x3), where x1, x2, x3 are positive real numbers for which x1 + x2 + x3 = 9. Then value of log2(m3) + log3(M2) is _______
14. Let a1, a2, a3,… be a sequence of positive integers in arithmetic progression with common difference 2. Also, let b1, b2, b3,… be a sequence of positive integers in geometric progression with common ratio 2. If a1 = b1 = c, then the number of all possible values of c, for which the equality 2(a1 + a2 + ⋯ + an) = b1 + b2 + ⋯ + bn holds for some positive integer n, is _____
15. Let f : [0, 2] → R be the function defined by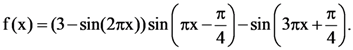 
If α, β ∈ [0, 2] are such that {x ∈ [0, 2] : f(x) ≥ 0} = [α, β], then the value of β – α is ________
16. In a triangle PQR, let 
If 
and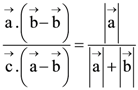 
Then the value of 
is _________
17. For a polynomial g(x) with real coefficients, let 𝑚𝑔 denote the number of distinct real roots of g(x). Suppose S is the set of polynomials with real coefficients defined by S = {(x2 – 1)2(a0 + a1x + a2x2 + a3x3) : a0, a1, a2, a3 ∈ R}
For a polynomial f, let f’ and f” denote its first and second order derivatives, respectively.
Then the minimum possible value of (mf‘ + mf”), where f ∈ S, is _____
18. Let e denote the base of the natural logarithm. The value of the real number a for which the right-hand limit is equal to a nonzero real number, is _____
Latest Govt Job & Exam Updates: