JEE (Advanced) Examination – 2022
(Held on Sunday 28th August, 2022)
PAPER-2
PHYSICS
SECTION-1 : (Maximum Marks : 24)
• This section contains EIGHT (08) questions.
• The answer to each question is a SINGLE DIGIT INTEGER ranging from 0 to 9, BOTH INCLUSIVE.
• For each question, enter the correct integer corresponding to the answer using the mouse and the on-screen virtual numeric keypad in the place designated to enter the answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +3 If ONLY the correct integer is entered;
Zero Marks : 0 If the question is unanswered;
Negative Marks : −1 In all other cases.
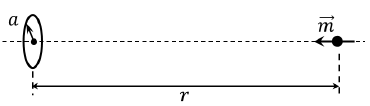
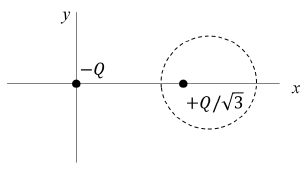
1. The particle of mass 1 kg is subjected to a force which depends on the position as with k = 1 kgs−2. At time t = 0, the particle’s position
and its velocity
Let vx and vy denote the x and the y components of the particle’s velocity, respectively. Ignore gravity. When z = 0.5 m, the value of (x vy – y vx) is ______ m2s−1.
2. In a radioactive decay chain reaction, nucleus decays into
The ratio of the number of α to number of β− particles emitted in this process is _____.
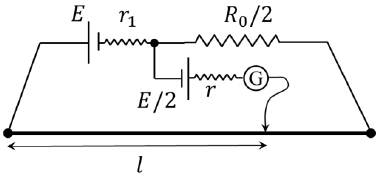
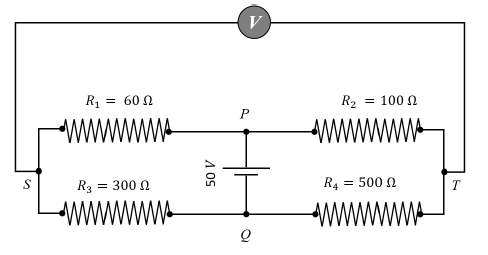
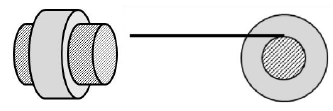
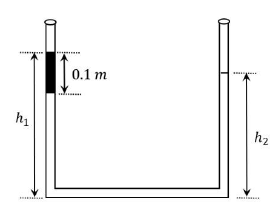
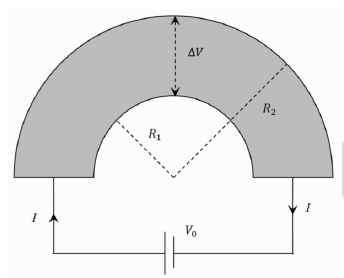
3. Two resistances R1 = XΩ and R2 = 1Ω are connected to a wire AB of uniform resistivity, as shown in the figure. The radius of the wire varies linearly along its axis from 0.2 mm at A to 1 mm at B. A galvanometer (G) connected to the center of the wire, 50 cm from each end along its axis, shows zero deflection when A and B are connected to a battery. The value of X is ______.
4. In a particular system of units, a physical quantity can be expressed in terms of the electric charge e, electron mass me, Planck’s constant h, and Coulomb’s constant where ∈0 is the permittivity of vacuum. In terms of these physical constants, the dimension of the magnetic field is [B] = [e]α[me]β [h]γ [k]δ. The value of α + β + γ + δ is _______.
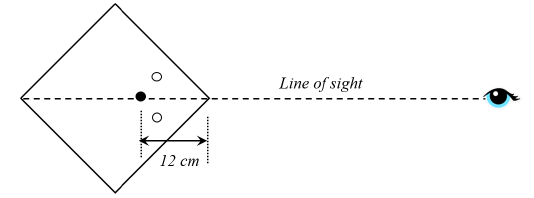
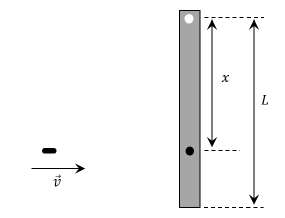
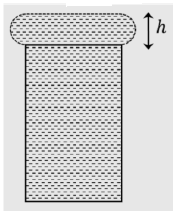
5. Consider a configuration of n identical units, each consisting of three layers. The first layer is a column of air of height h = 1/3 cm, and the second and third layers are of equal thickness and refractive indices
respectively. A light source O is placed on the top of the first unit, as shown in the figure. A ray of light from O is incident on the second layer of the first unit at an angle of θ = 60° to the normal. For a specific value of n, the ray of light emerges from the bottom of the configuration at a distance
as shown in the figure. The value of n is _______.
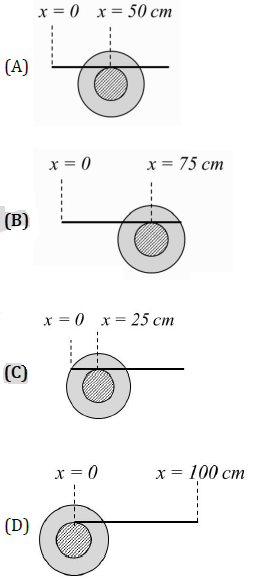
6. A charge q is surrounded by a closed surface consisting of an inverted cone of height h and base radius R, and a hemisphere of radius R as shown in the figure. The electric flux through the conical surface is The value of n is ______.
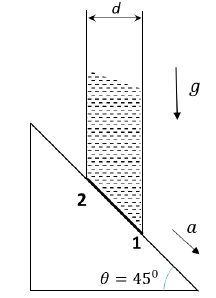
7. On a frictionless horizontal plane, a bob of mass m = 0.1 kg is attached to a spring with natural length l0 = 0.1 m. The spring constant is k1 = 0.009 Nm−1 when the length of the spring l > l0 and is k2 = 0.016 Nm−1 when l < l0. Initially the bob is released from l = 0.15 m. Assume that Hooke’s law remains valid throughout the motion. If the time period of the full oscillation is T = (nπ) s, then the integer closest to n is ______.
8. An object and a concave mirror of focal length f = 10 cm both move along the principal axis of the mirror with constant speeds. The object moves with speed V0 = 15 cm s−1 towards the mirror with respect to a laboratory frame. The distance between the object and the mirror at a given moment is denoted by u. When u = 30 cm, the speed of the mirror Vm is such that image is instantaneously at rest with respect to the laboratory frame, and the object forms a real image. The magnitude of Vm is _____ cm s−1.
SECTION-2: (Maximum Marks : 24)
• This section contains SIX (06) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONE OR MORE THAN ONE of these four option(s) is (are) correct answer(s).
• For each question, choose the option(s) corresponding to (all) the correct answer(s).
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 ONLY in (all) the correct option(s) is(are) chosen;
Partial Marks : +3 If all the four options are correct but ONLY three options are chosen;
Partial Marks : +2 If three or more options are correct but ONLY two options are chosen, both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If unanswered;
Negative Marks : −2 In all other cases.
9. In the figure, the inner (shaded) region A represents a sphere of radius rA = 1, within which the electrostatic charge density varies with the radial distance r from the center as ρA = kr, where k is positive. In the spherical shell B of outer radius rB, the electrostatic charge density varies as ρB = 2k/r. Assume that dimensions are taken care of. All physical quantities are in their SI units.
Which of the following statement(s) is(are) correct?
(A) If then the electric field is zero everywhere outside B.
(B) If rB = 3/2, then the electric potential just outside B is k/∈0.
(C) If rB = 2, then the total charge of the configuration is 15πk.
(D) If rB = 5/2, then the magnitude of the electric field just outside B is 13πk/∈0.
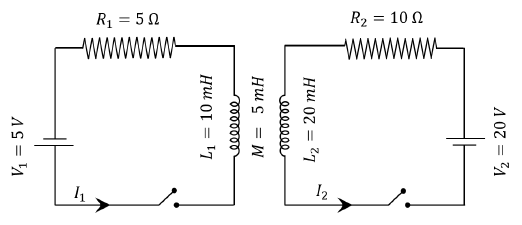
10. In Circuit-1 and Circuit-2 shown in the figures, R1 = 1 Ω, R2 = 2 Ω and R3 = 3 Ω, P1 and P2 are the power dissipations in Circuit-1 and Circuit-2 when the switches S1 and S2 are in open conditions, respectively.
Q1 and Q2 are the power dissipations in Circuit-1 and Circuit-2 when the switches S1 and S2 are in closed conditions, respectively.
Which of the following statement(s) is(are) correct?
(A) When a voltage source of 6 V is connected across A and B in both circuits, P1 < P2.
(B) When a constant current source of 2 Amp is connected across A and B in both circuits, P1 > P2.
(C) When a voltage source of 6 V is connected across A and B in Circuit-1, Q1 > P1.
(D) When a constant current source of 2 Amp is connected across A and B in both circuits, Q2 < Q1
11. A bubble has surface tension S. The ideal gas inside the bubble has ratio of specific heats γ = 5/3. The bubble is exposed to the atmosphere and it always retains its spherical shape. When the atmospheric pressure is Pa1, the radius of the bubble is found to be r1 and the temperature of the enclosed gas is T1. When the atmospheric pressure is Pa2, the radius of the bubble and the temperature of the enclosed gas are r2 and T2, respectively.
Which of the following statement(s) is(are) correct?
(A) If the surface of the bubble is a perfect heat insulator, then
(B) If the surface of the bubble is a perfect heat insulator, then the total internal energy of the bubble including its surface energy does not change with the external atmospheric pressure.
(C) If the surface of the bubble is a perfect heat conductor and the change in atmospheric temperature is negligible, then
(D) If the surface of the bubble is a perfect heat insulator, then
12. A disk of radius R with uniform positive charge density σ is placed on the xy plane with its center at the origin. The Coulomb potential along the z-axis is
A particle of positive charge q is placed initially at rest at a point on the z axis with z = z0 and z0 > 0. In addition to the Coulomb force, the particle experiences a vertical force Which of the following statement(s) is(are) correct?
(A) For the particle reaches the origin.
(B) For the particle reaches the origin.
(C) For the particle returns back to z = z0.
(D) For β > 1 and z0 > 0, the particle always reaches the origin.
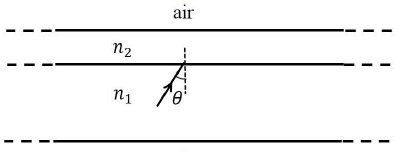
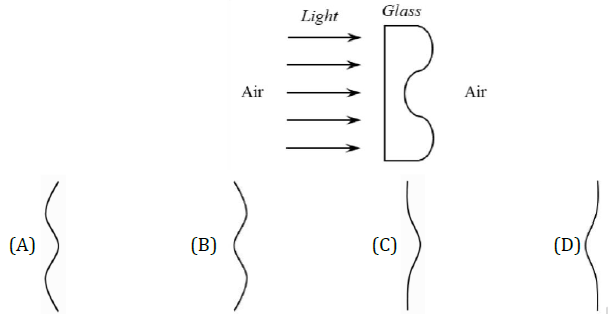
13. A double slit setup is shown in the figure. One of the slits is in medium 2 of refractive index n2. The other slit is at the interface of this medium with another medium 1 of refractive index n1(≠ n2). The line joining the slits is perpendicular to the interface and the distance between the slits is d. The slit widths are much smaller than d. A monochromatic parallel beam of light is incident on the slits from medium 1. A detector is placed in medium 2 at a large distance from the slits, and at an angle θ from the line joining them, so that θ equals the angle of refraction of the beam. Consider two approximately parallel rays from the slits received by the detector.
Which of the following statement(s) is(are) correct?
(A) The phase difference between the two rays is independent of d.
(B) The two rays interfere constructively at the detector.
(C) The phase difference between the two rays depends on n1 but is independent of n2.
(D) The phase difference between the two rays vanishes only for certain values of d and the angle of incidence of the beam, with θ being the corresponding angle of refraction.
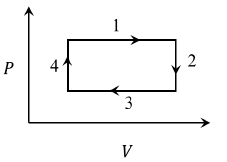
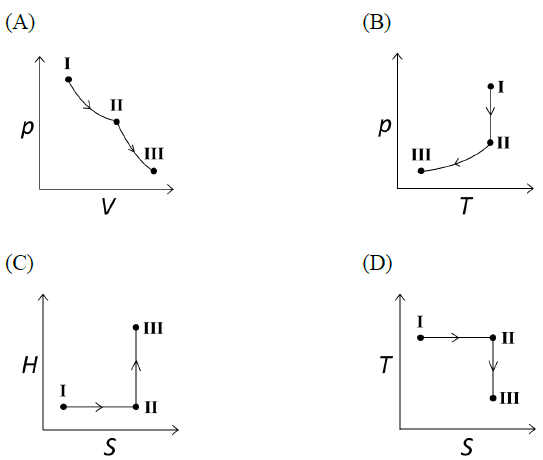
14. In the given P-V diagram, a monoatomic gas (γ = 5/3) is first compressed adiabatically from state A to state B. Then it expands isothermally from state B to state C. [Given : (1/3)6 = 0.5, ln 2 = 0.7].
Which of the following statement(s) is(are) correct?
(A) The magnitude of the total work done in the process A → B → C is 144 kJ.
(B) The magnitude of the work done in the process B → C is 84 kJ.
(C) The magnitude of the work done in the process A → B is 60 kJ.
(D) The magnitude of the work done in the process C → A is zero.
SECTION-3: (Maximum Marks : 12)
• This section contains FOUR (04) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONLY ONE of these four options is the correct answer.
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen:
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered);
Negative Marks : −1 In all other cases.
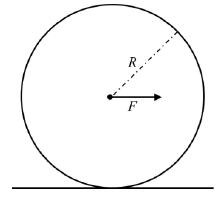
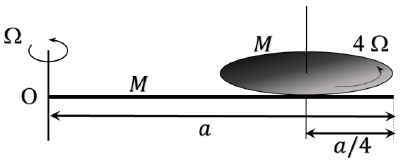
15. A flat surface of a thin uniform disk A of radius R is glued to a horizontal table. Another thin uniform disk B of mass M and with the same radius R rolls without slipping on the circumference of A, as shown in the figure. A flat surface of B also lies on the plane to the table. The center of mass of B has fixed angular speed ω about the vertical axis passing through the center of A. The angular momentum of B is nMωR2 with respect to the center of A. Which of the following is the value of n?
(A) 2
(B) 5
(C) 7/2
(D) 9/2
16. When light of a given wavelength is incident on a metallic surface, the minimum potential needed to stop the emitted photoelectrons is 6.0 V. This potential drops to 0.6 V if another source with wavelength four times that of the first one and intensity half of the first one is used. What are the wavelength of the first source and the work function of the metal, respectively?
(A) 1.72 × 10−7 m, 1.20 eV
(B) 1.72 × 10−7 m, 5.60 eV
(C) 3.78 × 10−7 m, 5.60 eV
(D) 3.78 × 10−7 m, 1.20 eV
17. Area of the cross-section of a wire is measured using a screw gauge. The pitch of the main scale is 0.5 mm. The circular scale has 100 divisions and for one full rotation of the circular scale, the main scale shifts by two divisions. The measured readings are listed below.
What are the diameter and cross-sectional area of the wire measured using the screw gauge?
(A) 2.22 ± 0.02 mm, π(1.23 ± 0.02) mm2
(B) 2.22 ± 0.01 mm, π(1.23 ± 0.01) mm2
(C) 2.14 ± 0.02 mm, π(1.14 ± 0.02) mm2
(D) 2.14 ± 0.01 mm, π(1.23 ± 0.01) mm2
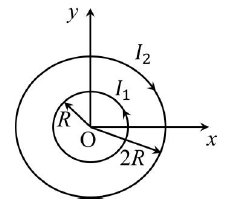
18. Which one of the following options represents the magnetic field at O due to the current flowing in the given wire segments lying on the xy plane?
CHEMISTRY
SECTION-1 : (Maximum Marks : 24)
• This section contains EIGHT (08) questions.
• The answer to each question is a SINGLE DIGIT INTEGER ranging from 0 to 9, BOTH INCLUSIVE.
• For each question, enter the correct integer corresponding to the answer using the mouse and the on-screen virtual numeric keypad in the place designated to enter the answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +3 If ONLY the correct integer is entered;
Zero Marks : 0 If the question is unanswered;
Negative Marks : −1 In all other cases.
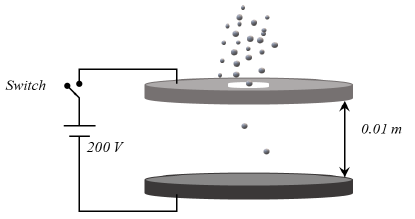
1. Concentration of H2SO4 and Na2SO4 in a solution is 1 M and 1.8 × 10–2 M, respectively. Molar solubility of PbSO4 in the same solution is X × 10–Y M (expressed in scientific notation). The value of Y is _________.
[Given: Solubility product of PbSO4 (Ksp) = 1.6 × 10–8. For H2SO4, Ka1 is very large and Ka2 = 1.2 × 10–2]
2. An aqueous solution is prepared by dissolving 0.1 mol of an ionic salt in 1.8 kg of water at 35 ºC. The salt remains 90% dissociated in the solution. The vapour pressure of the solution is 59.724 mm of Hg. Vapour pressure of water at 35 ºC is 60.000 mm of Hg. The number of ions present per formula unit of the ionic salt is _______.
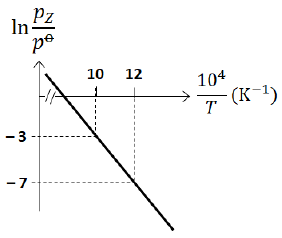
3. Consider the strong electrolytes ZmXn, UmYp and VmXn. Limiting molar conductivity (⋀0) of UmYp and VmXn are 250 and 440 S cm2 mol–1, respectively. The value of (m + n + p) is _______.
Given:
The plot of molar conductivity (⋀) of ZmXn vs c1/2 is given below.
4. The reaction of Xe and O2F2 gives a Xe compound P. The number of moles of HF produced by the complete hydrolysis of 1 mol of P is _______.
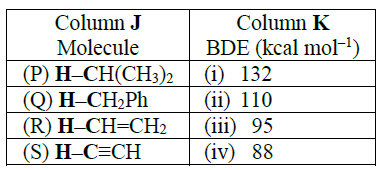
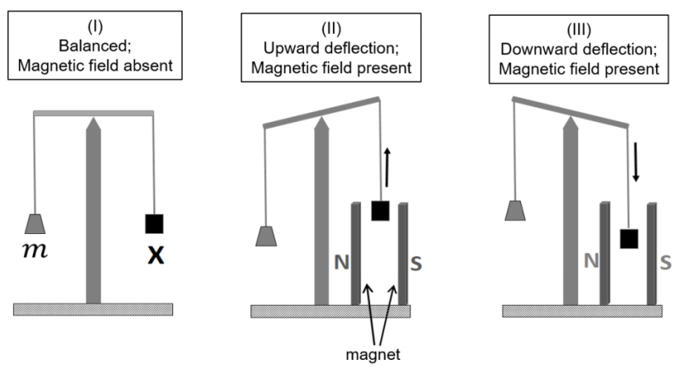
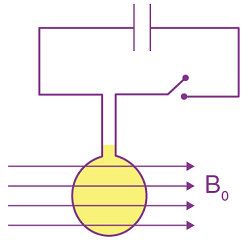
5. Thermal decomposition of AgNO3 produces two paramagnetic gases. The total number of electrons present in the antibonding molecular orbitals of the gas that has the higher number of unpaired electrons is _______.
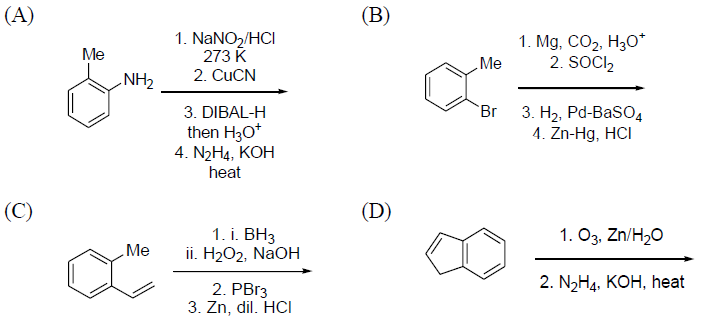
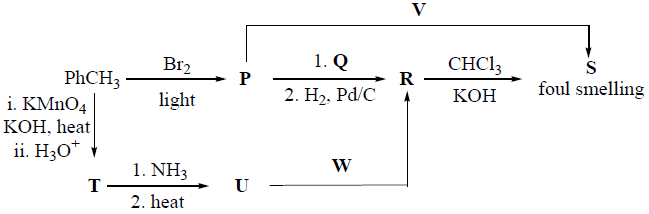
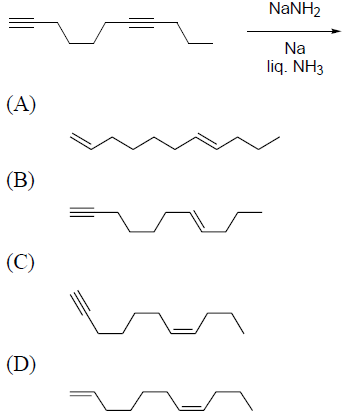
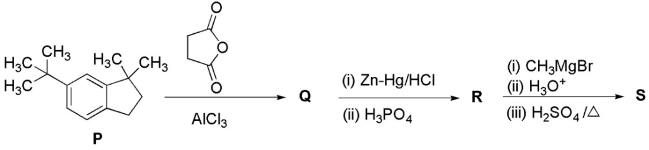
6. The number of isomeric tetraenes (NOT containing sp-hybridized carbon atoms) that can be formed from the following reaction sequence is ________.
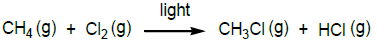
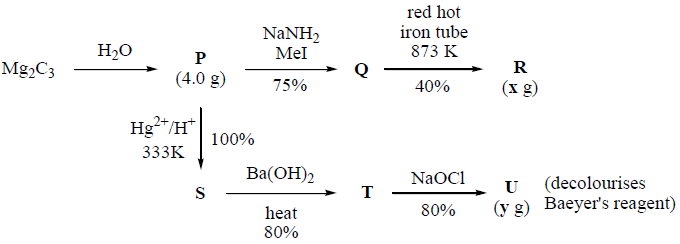
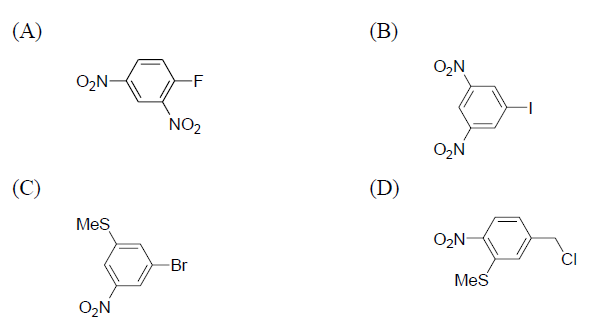
7. The number of –CH2-(methylene) groups in the product formed from the following reaction sequence is ________.
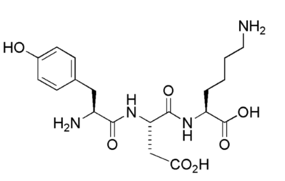
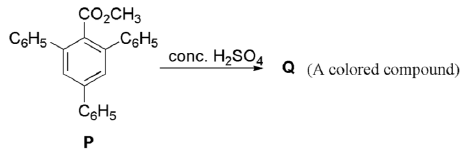
8. The total number of chiral molecules formed from one molecule of P on complete ozonolysis (O3, Zn/H2O) is ________.
SECTION-2: (Maximum Marks : 24)
• This section contains SIX (06) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONE OR MORE THAN ONE of these four option(s) is (are) correct answer(s).
• For each question, choose the option(s) corresponding to (all) the correct answer(s).
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 ONLY in (all) the correct option(s) is(are) chosen;
Partial Marks : +3 If all the four options are correct but ONLY three options are chosen;
Partial Marks : +2 If three or more options are correct but ONLY two options are chosen, both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If unanswered;
Negative Marks : −2 In all other cases.
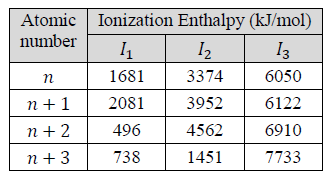
9. To check the principle of multiple proportions, a series of pure binary compounds (PmQn) were analyzed and their composition is tabulated below. The correct option(s) is(are)
(A) If empirical formula of compound 3 is P3Q4, then the empirical formula of compound 2 is P3Q5.
(B) If empirical formula of compound 3 is P3Q2 and atomic weight of element P is 20, then the atomic weight of Q is 45.
(C) If empirical formula of compound 2 is PQ, then the empirical formula of the compound 1 is P5Q4.
(D) If atomic weight of P and Q are 70 and 35, respectively, then the empirical formula of compound 1 is P2Q.
10. The correct option(s) about entropy (S) is(are)
[R = gas constant, F = Faraday constant, T = Temperature]
(A) For the reaction, M(s) + 2H+(aq) → H2(g) + M2+(aq), if then the entropy change of the reaction is R (assume that entropy and internal energy changes are temperature independent).
(B) The cell reaction, Pt(s) | H2(g, 1bar) | H+(aq, 0.01M) || H+(aq, 0.1M) | H2(g, 1bar) | Pt(s), is an entropy driven process.
(C) For racemization of an optically active compound, ∆S > 0.
(D) ∆S > 0, for [Ni(H2O)6]2+ + 3 en → [Ni(en)3]2+ + 6H2O (where en = ethylenediamine).
11. The compound(s) which react(s) with NH3 to give boron nitride (BN) is(are)
(A) B
(B) B2H6
(C) B2O3
(D) HBF4
12. The correct option(s) related to the extraction of iron from its ore in the blast furnace operating in the temperature range 900 – 1500 K is(are)
(A) Limestone is used to remove silicate impurity.
(B) Pig iron obtained from blast furnace contains about 4% carbon.
(C) Coke (C) converts CO2 to CO.
(D) Exhaust gases consist of NO2 and CO.
13. Considering the following reaction sequence, the correct statement(s) is(are)
(A) Compounds P and Q are carboxylic acids.
(B) Compound S decolorizes bromine water.
(C) Compounds P and S react with hydroxylamine to give the corresponding oximes.
(D) Compound R reacts with dialkylcadmium to give the corresponding tertiary alcohol.
14. Among the following, the correct statement(s) about polymers is(are)
(A) The polymerization of chloroprene gives natural rubber.
(B) Teflon is prepared from tetrafluoroethene by heating it with persulphate catalyst at high pressures.
(C) PVC are thermoplastic polymers.
(D) Ethene at 350-570 K temperature and 1000-2000 atm pressure in the presence of a peroxide initiator yields high density polythene.
SECTION-3: (Maximum Marks : 12)
• This section contains FOUR (04) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONLY ONE of these four options is the correct answer.
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen:
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered);
Negative Marks : −1 In all other cases.
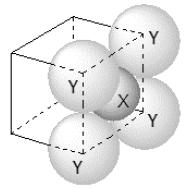
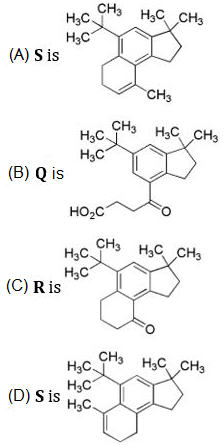
15. Atom X occupies the fcc lattice sites as well as alternate tetrahedral voids of the same lattice. The packing efficiency (in %) of the resultant solid is closest to
(A) 25
(B) 35
(C) 55
(D) 75
16. The reaction of HClO3 with HCl gives a paramagnetic gas, which upon reaction with O3 produces
(A) Cl2O
(B) ClO2
(C) Cl2O6
(D) Cl2O7
17. The reaction Pb(NO3)2 and NaCl in water produces a precipitate that dissolves upon the addition of HCl of appropriate concentration. The dissolution of the precipitate is due to the formation of
(A) PbCl2
(B) PbCl4
(C) [PbCl4]2−
(D) [PbCl6]2−
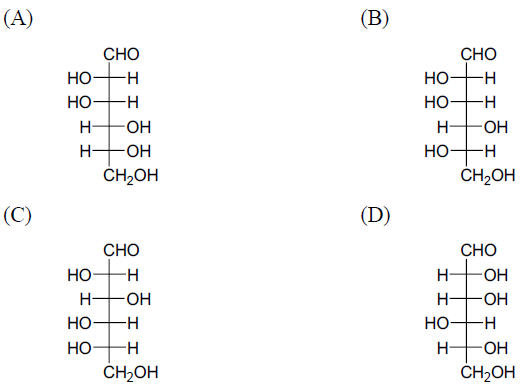
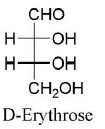
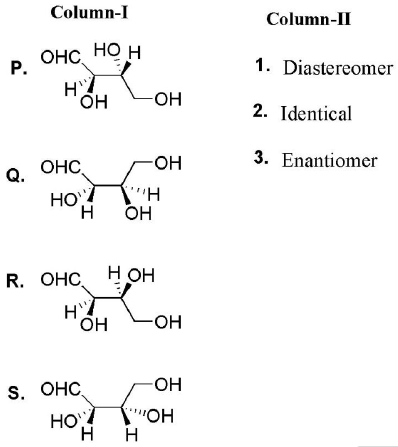
18. Treatment of D- glucose with aqueous NaOH results in a mixture of monosaccharides, which are
MATHEMATICS
SECTION-1 : (Maximum Marks : 24)
• This section contains EIGHT (08) questions.
• The answer to each question is a SINGLE DIGIT INTEGER ranging from 0 to 9, BOTH INCLUSIVE.
• For each question, enter the correct integer corresponding to the answer using the mouse and the on-screen virtual numeric keypad in the place designated to enter the answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full marks : +3 If ONLY the correct integer is entered;
Zero Marks : 0 If the question is unanswered;
Negative Marks : −1 In all other cases.
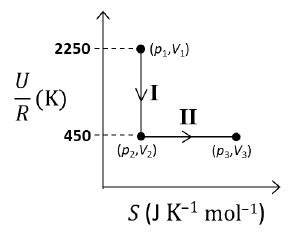
1. Let α and β be real numbers such that If
then the greatest integer less than or equal to
is _______.
2. If y(x) is the solution of the differential equation xdy – (y2 – 4y)dx = 0 for x > 0, y(1) = 2, and the slope of the curve y = y(x) is never zero, then the value of 10y(√2) is ______.
3. The greatest integer less than or equal to is ______.
4. The product of all positive real values of x satisfying the equation is ___________.
5. If
Then the value of 6β is ______.
6. Let β be a real number. Consider the matrix
If A7 – (β – 1)A6 – βA5 is a singular matrix, then the value of 9β is _______.
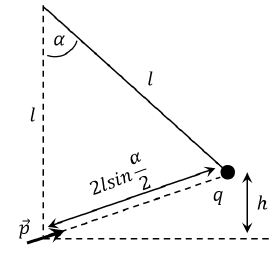
7. Consider the hyperbola with foci at S and S1, where S lies on the positive x-axis. Let P be a point on the hyperbola, in the first quadrant. Let ∠SPS1 = α, with α < π/2. The straight line passing through the point S and having the same slope as that of the tangent at P to the hyperbola, intersects the straight line S1P at P1. Let δ be the distance of P from the straight line SP1, and β= S1 Then the greatest integer less than or equal to
is _______.
8. Consider the functions f , g : ℝ → ℝ defined by
and
If α is the area of the region then the value of 9α is ______.
SECTION-2: (Maximum Marks : 24)
• This section contains SIX (06) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONE OR MORE THAN ONE of these four option(s) is (are) correct answer(s).
• For each question, choose the option(s) corresponding to (all) the correct answer(s).
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +4 ONLY in (all) the correct option(s) is(are) chosen;
Partial Marks : +3 If all the four options are correct but ONLY three options are chosen;
Partial Marks : +2 If three or more options are correct but ONLY two options are chosen, both of which are correct;
Partial Marks : +1 If two or more options are correct but ONLY one option is chosen and it is a correct option;
Zero Marks : 0 If unanswered;
Negative Marks : −2 In all other cases.
9. Let PQRS be a quadrilateral in a plane, where QR = 1, ∠PQR = ∠QRS = 70°, ∠PQS = 15° and ∠PRS = 40°. If ∠RPS = θ°, PQ = α and PS = β, then the interval(s) that contain(s) the value of 4αβ sin θ° is/are
(A) (0, √2)
(B) (1, 2)
(C) (√2, 3)
(D) (2√2, 3√2)
10. Let
Let g : [0, 1] → ℝ be the function defined by g(x) = 2αx + 2α(1 – x)
Then, which of the following statements is/are TRUE?
(A) The minimum value of g(x) is 27/6
(B) The maximum value of g(x) is 1 + 21/3
(C) The function g(x) attains its maximum at more than one point
(D) The function g(x) attains its minimum at more than one point
11. Let denote the complex conjugate of a complex number z. If z is a non-zero complex number for which both real and imaginary parts of
are integers, then which of the following is/are possible value(s) of |z|?
12. Let G be a circle of radius R > 0. Let G1, G2…,Gn be n circles of equal radius r > 0. Suppose each of the n circles G1, G2…,Gn touches the circle G externally. Also, for i = 1, 2,…, n – 1, the circle Gi touches Gi+1 externally, and Gn touches G1 Then, which of the following statements is/are TRUE?
(A) If n = 4, then (√2 – 1) r < R
(B) If n = 5, then r < R
(C) If n = 8, then (√2 – 1) r < R
(D) If n = 12, then √2(√3 + 1) r > R
13. Let be the unit vectors along the three positive coordinate axes. Let
be three vectors such that b2b3 > 0, and
Then, which of the following is/are TRUE?
14. For x ∈ ℝ, let the function y(x) be the solution of the differential equation
Then, which of the following statements is/are TRUE?
(A) y(x) is an increasing function
(B) y(x) is a decreasing function
(C) There exists a real number β such that the line y = β intersects the curve y = y many points.
(D) y(x) is a periodic function
SECTION-3: (Maximum Marks : 12)
• This section contains FOUR (04) questions.
• Each question has FOUR options (A), (B), (C) and (D). ONLY ONE of these four options is the correct answer.
• For each question, choose the option corresponding to the correct answer.
• Answer to each question will be evaluated according to the following marking scheme:
Full Marks : +3 If ONLY the correct option is chosen:
Zero Marks : 0 If none of the options is chosen (i.e. the question is unanswered);
Negative Marks : −1 In all other cases.
15. Consider 4 boxes, where each box contains 3 red balls and 2 blue balls. Assume that distinct. In how many different ways can 10 balls be chosen from these 4 boxes so box at least one red ball and one blue ball are chosen?
(A) 21816
(B) 85536
(C) 12096
(D) 156816
16. If then which of the following matrices is equal to M2022?
17. Suppose that
Box-I contains 8 red, 3 blue and 5 green balls,
Box-II contains 24 red, 9 blue and 15 green balls,
Box-III contains 1 blue, 12 green and 3 yellow balls,
Box-IV contains 10 green, 16 orange and 6 white balls.
A ball is chosen randomly from Box-I ; call this ball b. If b is red then a ball is chosen randomly from Box-II, if b is blue then a ball is chosen randomly from Box-III, and if b is green then a ball is chosen randomly from Box-IV. The conditional probability of the event ‘one of the chosen balls is white’ given that the event ꞌat least one of the chosen balls is greenꞌ has happened, is equal to
(A) 15/256
(B) 3/16
(C) 5/52
(D) 1/8
18. For positive integer n, define
Then, the value of is equal to

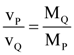
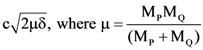
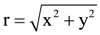

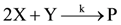
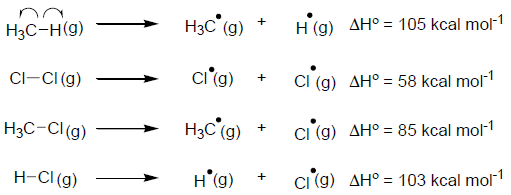
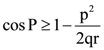
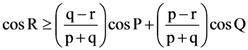
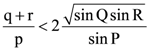
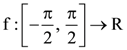 be a continuous function such that
be a continuous function such that 
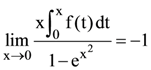
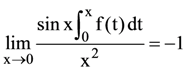
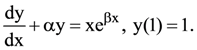 Let S = {yα,β (x), α, β ∈ R } . Then which of the following functions belong(s) to the set S?
Let S = {yα,β (x), α, β ∈ R } . Then which of the following functions belong(s) to the set S?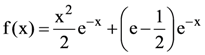
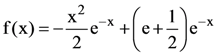
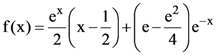
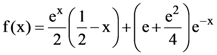
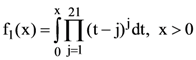
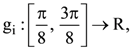 i = 1, 2, and
i = 1, 2, and 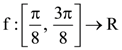 be functions such that g1(x) = 1, g2(x) = |4x – π| and f(x) = sin2 x, for all
be functions such that g1(x) = 1, g2(x) = |4x – π| and f(x) = sin2 x, for all 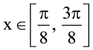
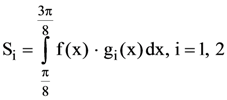
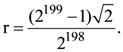 The number of all those circles Dn that are inside M is;
The number of all those circles Dn that are inside M is;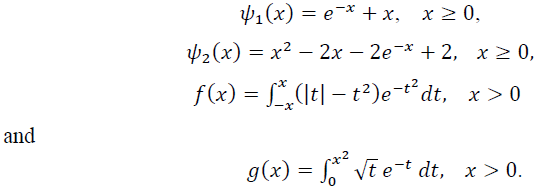
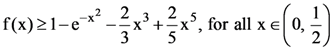
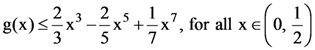
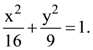 For any three distinct points P, Q and Q’ on E, let M (P, Q) be the mid-point of the line segment joining P and Q, and M(P, Q’) be the mid-point of the line segment joining P and Q’. Then the maximum possible value of the distance between M (P, Q) and M(P, Q’), as P, Q and Q’ vary on E, is
For any three distinct points P, Q and Q’ on E, let M (P, Q) be the mid-point of the line segment joining P and Q, and M(P, Q’) be the mid-point of the line segment joining P and Q’. Then the maximum possible value of the distance between M (P, Q) and M(P, Q’), as P, Q and Q’ vary on E, is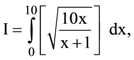 then the value of 9I is ______.
then the value of 9I is ______.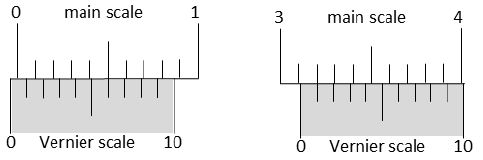
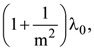 where λ0 is the shortest wavelength of Lyman series and m is an integer
where λ0 is the shortest wavelength of Lyman series and m is an integer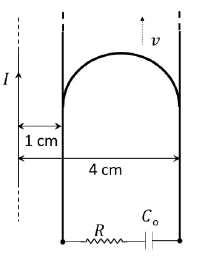
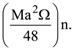
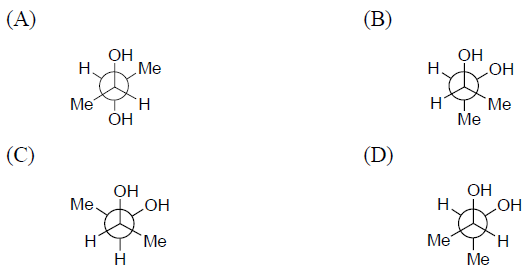
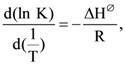 where the equilibrium constant,
where the equilibrium constant, 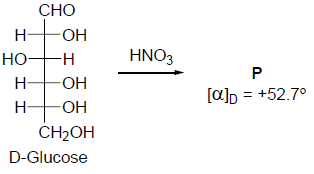

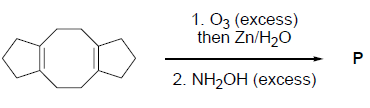
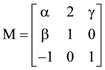
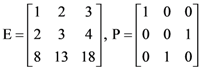
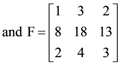

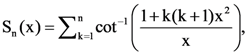
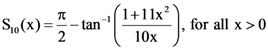
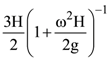
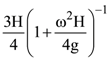

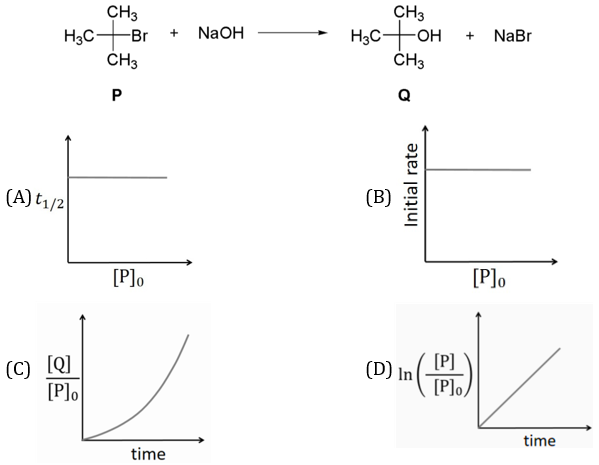
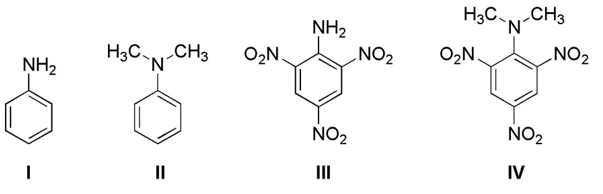
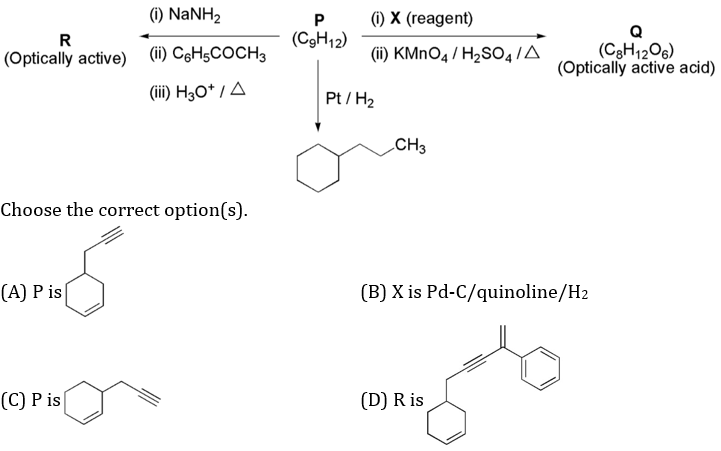
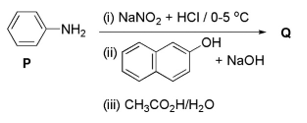
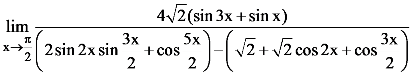 is ______
is ______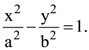 Suppose the tangent to the hyperbola at P passes through the point (1, 0), and suppose the normal to the hyperbola at P cuts off equal intercepts on the coordinate axes. Let Δ denote the area of the triangle formed by the tangent at P, the normal at P and the x-axis. If e denotes the eccentricity of the hyperbola, then which of the following statements is/are TRUE?
Suppose the tangent to the hyperbola at P passes through the point (1, 0), and suppose the normal to the hyperbola at P cuts off equal intercepts on the coordinate axes. Let Δ denote the area of the triangle formed by the tangent at P, the normal at P and the x-axis. If e denotes the eccentricity of the hyperbola, then which of the following statements is/are TRUE?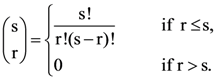
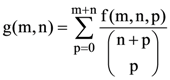
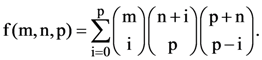
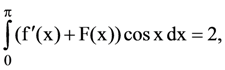 then the value of f(0) is _______
then the value of f(0) is _______
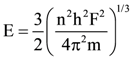
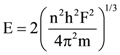
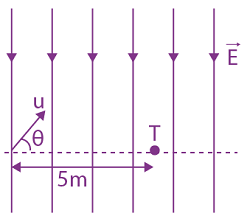
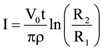
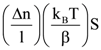
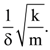 The value of δ is ______.
The value of δ is ______.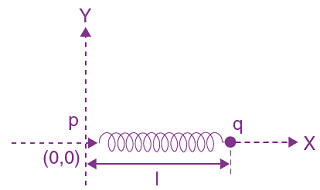
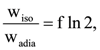 then f is ________.
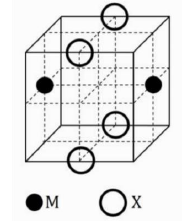
then f is ________.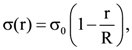 where 𝜎0 is a constant and r is the distance from the center of the disc. Electric flux through a large spherical surface that encloses the charged disc completely is ϕ0. Electric flux through another spherical surface of radius R/4 and concentric with the disc is ϕ. Then the ratio
where 𝜎0 is a constant and r is the distance from the center of the disc. Electric flux through a large spherical surface that encloses the charged disc completely is ϕ0. Electric flux through another spherical surface of radius R/4 and concentric with the disc is ϕ. Then the ratio 
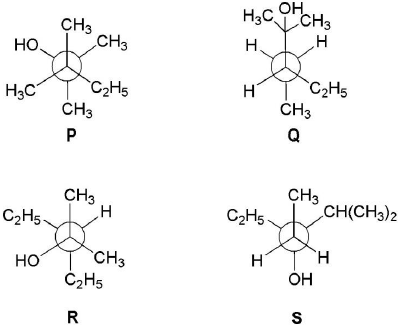
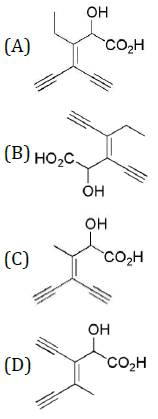
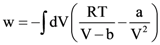
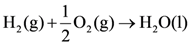
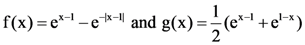
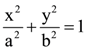 passes through the point𝑃. If the tangents to the parabola and the ellipse at the point P are perpendicular to each other, then the eccentricity of the ellipse is
passes through the point𝑃. If the tangents to the parabola and the ellipse at the point P are perpendicular to each other, then the eccentricity of the ellipse is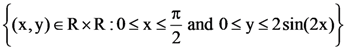 and having one side on the x-axis. The area of the rectangle which has the maximum perimeter among all such rectangles, is
and having one side on the x-axis. The area of the rectangle which has the maximum perimeter among all such rectangles, is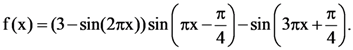
 and
and 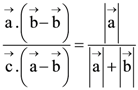
 is _________
is _________