Physics
1. A circular coil has moment of inertia 0.8 kg m2 around any diameter and is carrying current to produce a magnetic moment of 20 Am2. The coil is kept initially in a vertical position and it can rotate freely around a horizontal diameter. When a uniform magnetic field of 4 T is applied along the vertical, it starts rotating around its horizontal diameter. The angular speed the coil acquires after rotating by 60° will be:
(1) 10 π rad s–1
(2) 20 rad s–1
(3) 20 π rad s–1
(4) 10 rad s–1
2. A person pushes a box on a rough horizontal platform surface. He applies a force of 200 N over a distance of 15 m. Thereafter, he gets progressively tired and his applied force reduces linearly with distance to 100 N. The total distance through which the box has been moved is 30 m. What is the work done by the person during the total movement of the box?
(1) 5690 J
(2) 5250 J
(3) 2780 J
(4) 3280 J
3. Match the thermodynamic processes taking place in a system with the correct conditions. In the table: ∆Q is the heat supplied, ∆W is the work done and ∆U is change in internal energy of the system.
Process – Condition
(I) Adiabatic – (1) ∆W = 0
(II) Isothermal – (2) ∆ Q = 0
(III) Isochoric – (3) ∆U ≠0, ∆W ≠ 0, ∆Q ≠ 0
(IV) Isobaric – (4) ∆U = 0
(1) (I) – (1), (II) – (1), (III) – (2), (IV) – (3)
(2) (I) – (1), (II) – (2), (III) – (4), (IV) – (4)
(3) (I) – (2), (II) – (4), (III) – (1), (IV) – (3)
(4) (I) – (2), (II) – (1), (III) – (4), (IV) – (3)
4. The driver of a bus approaching a big wall notices that the frequency of his bus’s horn changes from 420 Hz to 490 Hz when he hears it after it gets reflected from the wall. Find the speed of the bus if speed of the sound is 330 ms–1.
(1) 81 kmh–1
(2) 91 kmh–1
(3) 71 kmh–1
(4) 61 kmh–1
5. A small ball of mass m is thrown upward with velocity u from the ground. The ball experiences a resistive force mkv2 where v is its speed. The maximum height attained by the ball is:
(1) 
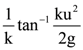
(2) 
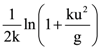
(3) 
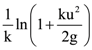
(4) 
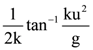
6. Consider two uniform discs of the same thickness and different radii R1= R and R2 =αR made of the same material. If the ratio of their moments of inertia I1 and I2, respectively, about their axes is I1: I2 = 1 : 16 then the value of α is
(1) √2
(2) 2
(3) 2√2
(4) 4
7. A series L-R circuit is connected to a battery of emf V. If the circuit is switched on at t =0, then the time at which the energy stored in the inductor reaches (1/n) times of its maximum value, is:
(1) 
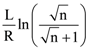
(2) 
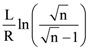
(3) 
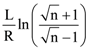
(4) 
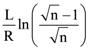
8. The electric field of a plane electromagnetic wave is given by 
Its magnetic field will be given by:
(1) 
(2) 
(3) 
(4) 
9. A cube of metal is subjected to a hydrostatic pressure of 4 GPa. The percentage change in the length of the side of the cube is close to: (Given bulk modulus of metal, B = 8 × 1010 Pa)
(1) 0.6
(2) 20
(3) 1.67
(4) 5
10. A paramagnetic sample shows a net magnetisation of 6 A/m when it is placed in an external magnetic field of 0.4 T at a temperature of 4 K. When the sample is placed in an external magnetic field of 0.3 T at a temperature of 24 K, then the magnetisation will be:
(1) 4 A/m
(2) 1 A/m
(3) 0.75 A/m
(4) 2.25 A/m
11. A body is moving in a low circular orbit about a planet of mass M and radius R. The radius of the orbit can be taken to be R itself. Then the ratio of the speed of this body in the orbit to the escape velocity from the planet is:
(1) 2
(2) √2
(3) 1
(4) 1/√2
12. A particle of charge q and mass m is subjected to an electric field E = E0 (1 – ax2) in the x-direction, where a and E0 are constants. Initially the particle was at rest at x = 0. Other than the initial position the kinetic energy of the particle becomes zero when the distance of the particle from the origin is:
(1) 
(2) a
(3) 
(4) 
13. A capacitor C is fully charged with voltage V0. After disconnecting the voltage source, it is connected in parallel with another uncharged capacitor of capacitance C/2. The energy loss in the process after the charge is distributed between the two capacitors is:
(1) 
(2) 
(3) 
(4) 
14. Find the Binding energy per nucleon for 
Mass of proton mp = 1.00783 U, mass of neutron mn = 1.00867 U and mass of tin nucleus mSn = 119.902199 U. (take 1U = 931 MeV)
(1) 8.0 MeV
(2) 9.0 MeV
(3) 7.5 MeV
(4) 8.5 MeV
15. The value of current i1 flowing from A to C in the circuit diagram is:
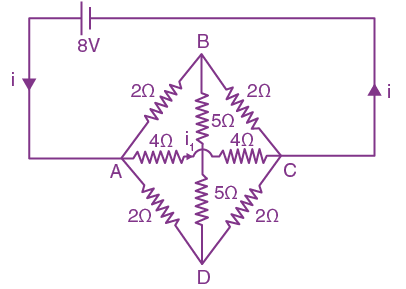
(1) 4 A
(2) 5 A
(3) 2 A
(4) 1 A
16. Two identical cylindrical vessels are kept on the ground and each contain the same liquid of density d. The area of the base of both vessels is S but the height of liquid in one vessel is x1 and in the other, x2. When both cylinders are connected through a pipe of negligible volume very close to the bottom, the liquid flows from one vessel to the other until it comes to equilibrium at a new height. The change in energy of the system in the process is:
(1) gdS(x2 + x1)2
(2) 
(3) 
(4) 
17. A quantity x is given by (IFv2/WL4) in terms of moment of inertia I, force F, velocity v, work W and Length L. The dimensional formula for x is same as that of:
(1) coefficient of viscosity
(2) energy density
(3) force constant
(4) planck’s constant
18. For a uniform rectangular sheet shown in the figure, the ratio of moments of inertia about the axes perpendicular to the sheet and passing through O (the centre of mass) and O’ (corner point) is:
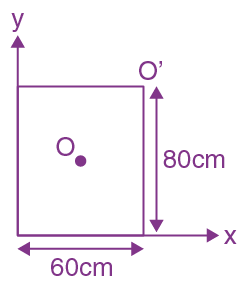
(1) 1/2
(2) 2/3
(3) 1/4
(4) 1/8
19. Identify the operation performed by the circuit given below:
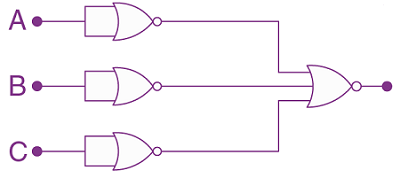
(1) NOT
(2) OR
(3) AND
(4) NAND
20. In a photoelectric effect experiment, the graph of stopping potential V versus reciprocal of wavelength obtained is shown in the figure. As the intensity of incident radiation is increased:
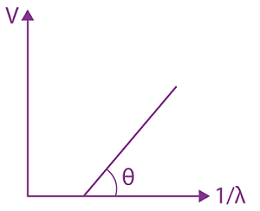
(1) Straight line shifts to right
(2) Straight line shifts to left
(3) Slope of the straight line get more steep
(4) Graph does not change
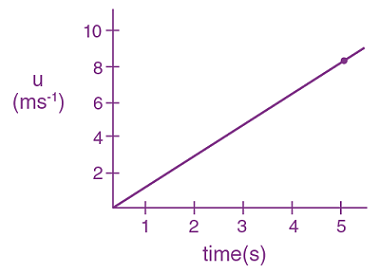
21. The speed verses time graph for a particle is shown in the figure. The distance travelled (in m) by the particle during the time interval t = 0 to t = 5 s will be________.
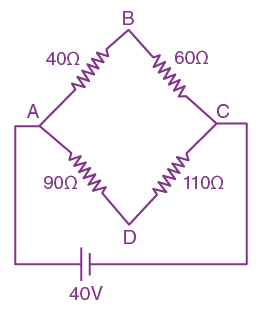
22. Four resistances 40 Ω, 60 Ω, 90 Ω and 110 Ω make the arms of a quadrilateral ABCD. Across AC is a battery of emf 40 V and internal resistance negligible. The potential difference across BD in V is _______.
23. The change in the magnitude of the volume of an ideal gas when a small additional pressure ∆P is applied at a constant temperature, is the same as the change when the temperature is reduced by a small quantity ∆T at constant pressure. The initial temperature and pressure of the gas were 300 K and 2 atm. respectively. If │∆T│= C│∆ P│ then value of C in (K/atm.) is _________.
24. Orange light of wavelength 6000 × 10–10 m illuminates a single slit of width 0.6 × 10–4 The maximum possible number of diffraction minima produced on both sides of the central maximum is ___________.
25. The distance between an object and a screen is 100 cm. A lens can produce real image of the object on the screen for two different positions between the screen and the object. The distance between these two positions is 40 cm. If the power of the lens is close to (N/100)D where N is an integer, the value of N is _________.
Chemistry
1. The reaction in which the hybridisation of the underlined atom is affected is:
2. The process that is NOT endothermic in nature is :
(1) H(g) + e− → H(g)−
(2) Na(g) → Na(g)− → e−
(3) Ar(g) + e− → Ar(g)−
(4) O(g)− + e− → O(g)2−
3. If the equilibrium constant for 
the equilibrium constant for A ⇌ P is:
4. A sample of red ink (a colloidal suspension) is prepared by mixing eosin dye, egg white, HCHO and water. The component which ensures the stability of the ink sample is :
(1) HCHO
(2) Water
(3) Eosin dye
(4) Egg white
5. The one that can exhibit the highest paramagnetic behaviour among the following is: gly = glycinato; bpy = 2, 2’-bipyridine
(1) [Ti (NH3)6]3+
(2) [Co (OX)2 (OH)2]−
(3) [Pd (gly)2]
(4) [Fe (en) (bpy) (NH3)2]2+
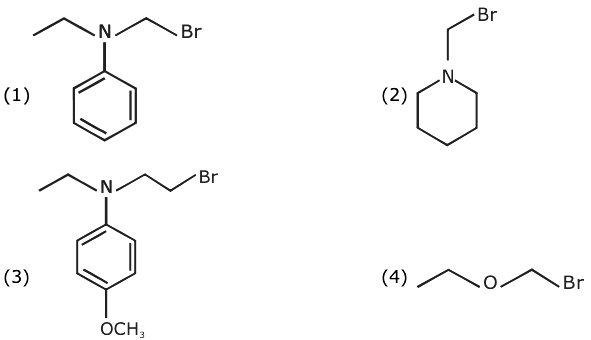
6. Which of the following compounds will form the precipitate with aq. AgNO3 solution most readily?
7. Five moles of an ideal gas at 1 bar and 298 K is expanded into the vacuum to double the volume. The work done is:
(1) zero
(2) CV[T2 – T1]
(3) −RT(V2 – V1)
(4) −RT ln(V2/V1)
8. 250 mL of a waste solution obtained from the workshop of a goldsmith contains 0.1 M AgNO3 and 0.1 M AuCl. The solution was electrolyzed at 2 V by passing a current of 1 A for 15 minutes. The metal/metals electrodeposited will be:
(1) Silver and gold in proportion to their atomic weights
(2) Silver and gold in equal mass proportion
(3) only silver
(4) only gold
9. The mechanism of action of “Terfenadine” (Seldane) is:
(1) Helps in the secretion of histamine
(2) Activates the histamine receptor
(3) Inhibits the secretion of histamine
(4) Inhibits the action of histamine receptor
10. The shortest wavelength of the H atom in the Lyman series is λ1. The longest wavelength in the Balmer series of He+ is:
(1) 9λ1/5
(2) 27λ1/5
(3) 36λ1/5
(4) 5λ1/9
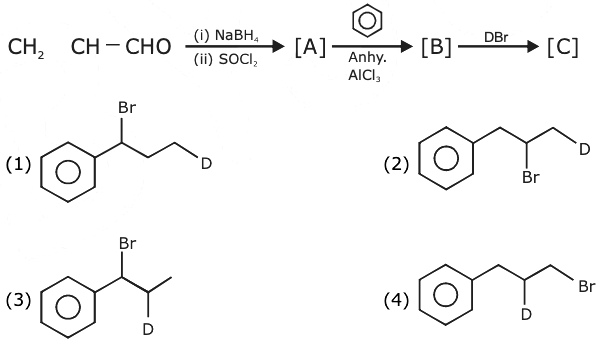
11. The major product [B] in the following reactions is:
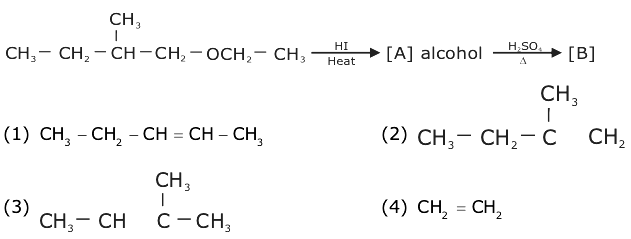
12. The major product [C] of the following reaction sequence will be:
13. The Crystal Field Stabilization Energy (CFSE) of [CoF3(H2O)3] (Δ0 < P) is:
(1) – 0.8Δ0
(2) – 0.8Δ0 + 2P
(3) – 0.4Δ0 + P
(4) – 0.4Δ0
14. Among the following compounds, which one has the shortest C – Cl bond?
15. The major product [R] in the following sequence of reactions is:
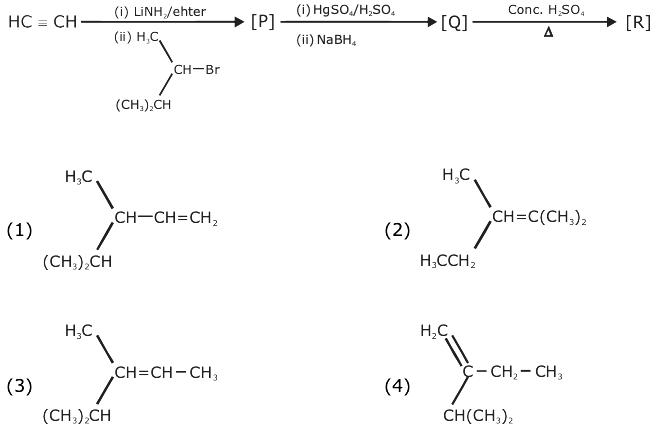
16. The molecule in which hybrid AOs involve only one d-orbital of the central atom is:
(1) [CrF6]3−
(2) XeF4
(3) BrF5
(4) [Ni(CN)4]2−
17. In the following reaction sequence, [C] is:
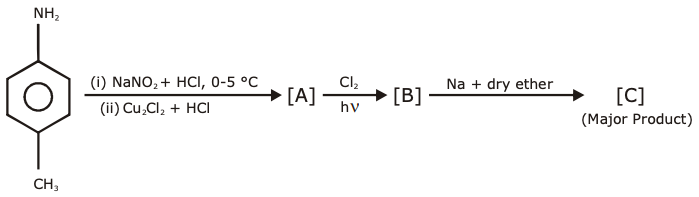
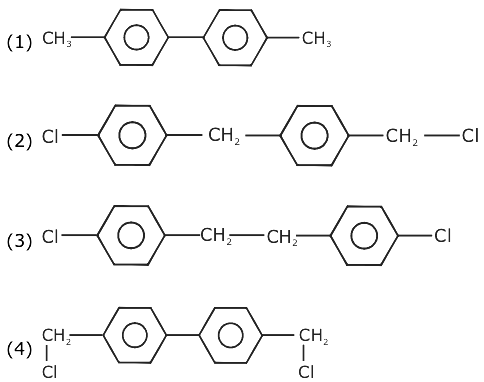
18. The processes of calcination and roasting in metallurgical industries, respectively, can lead to:
(1) Photochemical smog and ozone layer depletion
(2) Photochemical smog and global warming
(3) Global warming and photochemical smog
(4) Global warming and acid rain
19. The incorrect statement(s) among (a) – (c) is (are):
(a) W(VI) is more stable than Cr(VI).
(b) in the presence of HCl, permanganate titrations provide satisfactory results.
(c) some lanthanoid oxides can be used as phosphors.
(1) (a) only
(2) (b) and (c) only
(3) (a) and (b) only
(4) (b) only
20. An alkaline earth metal ‘M’ readily forms water-soluble sulphate and water-insoluble hydroxide. Its oxide MO is very stable to heat and does not have a rock-salt structure. M is :
(1) Ca
(2) Be
(3) Mg
(4) S
21. The osmotic pressure of a solution of NaCl is 0.10 atm and that of a glucose solution is 0.20 atm. The osmotic pressure of a solution formed by mixing 1 L of the sodium chloride solution with 2 L of the glucose solution is x × 10−3 x is _______. (nearest integer)
22. The number of molecules with energy greater than the threshold energy for a reaction increases fivefold by a rise of temperature from 27°C to 42 °C. Its energy of activation in J/mol is ________. (Take ln 5 = 1.6094 ; R = 8.314 J mol−1K−1)
23. A 100 mL solution was made by adding 1.43 g of Na2CO3 . xH2 The normality of the solution is 0.1 N. The value of x is ________. (The atomic mass of Na is 23 g/mol).
24. Consider the following equations:
2 Fe2+ + H2O2 → x A + y B (in basic medium)
2 MnO4− + 6 H+ + 5 H2O2 → x ‘C + y ‘D + z’E (in acidic medium).
The sum of the stoichiometric coefficients x, y, x’,y’ and z’ for products A, B, C, D and E, respectively, is _________.
25. The number of chiral centres present in threonine is ________.
Mathematics
1. Suppose the vectors x1, x2 and x3 are the solutions of the system of linear equations, Ax = b when the vector b on the right side is equal to b1, b2 and b3 If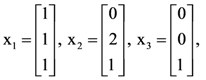 
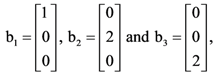then the determinant of A is equal to
(1) 2
(2) 1/2
(3) 3/2
(4) 4
2. If a and b are real numbers such that (2+α)4 = a + bα, where 
then a + b is equal to:
(1) 33
(2) 57
(3) 9
(4) 24
3. The distance of the point (1, −2, 3) from the plane x – y + z = 5 measured parallel to the line 
is:
(1) 1/7
(2) 7
(3) 7/5
(4) 1
4. Let f: (0, ∞) → (0, ∞) be a differentiable function such that f(1) = e and 
If f(x) = 1, then x is equal to:
(1) e
(2) 2e
(3) 1/e
(4) 1/2e
5. Contra positive of the statement :
‘If a function f is differentiable at a, then it is also continuous at a’, is:
(1) If a function f is not continuous at a, then it is not differentiable at a.
(2) If a function f is continuous at a, then it is differentiable at a.
(3) If a function f is continuous at a, then it is not differentiable at a.
(4) If a function f is not continuous at a, then it is differentiable at a.
6. The minimum value of 2sinx + 2cosx is:
(1) 21 – √2
(2) 21 – 1/√2
(3) 2– 1 + √2
(4) 2–1 + 1/√2
7. If the perpendicular bisector of the line segment joining the points P(1 ,4) and Q(k, 3) has y-intercept equal to −4, then a value of k is:
(1) −2
(2) √15
(3) √14
(4) −4
8. The area (in sq. units) of the largest rectangle ABCD whose vertices A and B lie on the x-axis and vertices C and D lie on the parabola, y = x2 − 1 below the x-axis, is:
(1) 2/3√3
(2) 4/3
(3) 1/3√3
(4) 4/3√3
9. The integral 
(2 sec2x ∙ sin2 3x + 3 tan x ∙ sin 6x) dx is equal to:
(1) 9/2
(2) −1/18
(3) −1/9
(4) 7/18
10. If the system of equations
x + y + z = 2
2x + 4y – z = 6
3x + 2y + λz = μ
has infinitely many solutions, then
(1) λ −2μ = -5
(2) 2λ + μ = 14
(3) λ + 2μ = 14
(4) 2λ – μ = 5
11. In a game two players A and B take turns in throwing a pair of fair dice starting with player A and total of scores on the two dice, in each throw is noted. A wins the game if he throws a total of 6 before B throws a total of 7 and B wins the game if he throws a total of 7 before A throws a total of six The game stops as soon as either of the players wins. The probability of A winning the game is :
(1) 5/31
(2) 31/61
(3) 30/61
(4) 5/6
12. If for some positive integer n, the coefficients of three consecutive terms in the binomial expansion of (1 + x)n+5 are in the ratio 5:10:14, then the largest coefficient in this expansion is :
(1) 792
(2) 252
(3) 462
(4) 330
13. The function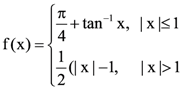 
is:
(1) both continuous and differentiable on R−{−1}
(2) continuous on R−{−1} and differentiable on R−{−1,1}
(3) continuous on R−{1} and differentiable on R−{−1, 1}
(4) both continuous and differentiable on R−{1}
14. The solution of the differential equation 
is: (where c is a constant of integration)
(1) x – loge(y + 3x) = C
(2) 
(3) x – 2loge(y + 3x) = C
(4) 
15. Let λ ≠ 0 be in R. If α and β are the roots of the equation, x2 – x + 2λ = 0 and α and γ are the roots of the equation, 3x2 − 10x + 27λ = 0, then βγ/λ is equal to:
(1) 27
(2) 9
(3) 18
(4) 36
16. The angle of elevation of a cloud C from a point P, 200 m above a still lake is 30°. If the angle of depression of the image of C in the lake from the point P is 60°, then PC (in m) is equal to :
(1) 200√3
(2) 400√3
(3) 400
(4) 100
17. Let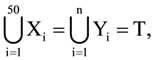 
where each Xi contains 10 elements and each Yi contains 5 elements. If each element of the set T is an element of exactly 20 of sets Xi ’s and exactly 6 of sets Yi’s, then n is equal to :
(1) 15
(2) 30
(3) 50
(4) 45
18. Let x = 4 be a directrix to an ellipse whose centre is at the origin and its eccentricity is 1/2. If P(1, β ), β > 0 is a point on this ellipse, then the equation of the normal to it at P is :
(1) 8x – 2y = 5
(2) 4x – 2y = 1
(3) 7x – 4y = 1
(4) 4x – 3y = 2
19. Let a1, a2, …, an be a given A.P. whose common difference is an integer and Sn = a1 + a2 + …. + an. If a1 = 1, an = 300 and 15 ≤ n ≤ 50, then the ordered pair (Sn−4, an−4) is equal to:
(1) (2480, 248)
(2) (2480, 249)
(3) (2490, 249)
(4) (2490, 248)
20. The circle passing through the intersection of the circles, x2 + y2 − 6x = 0 and x2 + y2 − 4y = 0, having its centre on the line, 2x − 3y + 12 = 0, also passes through the point:
(1) (−1, 3)
(2) (1, −3)
(3) (−3, 6)
(4) (−3, 1)
21. Let {x} and [x] denote the fractional part of x and the greatest integer ≤ x respectively of a real number x. If 
and 10(n2 – n), (n ∈ N, n > 1) are three consecutive terms of a G.P., then n is equal to______
22. A test consists of 6 multiple choice questions, each having 4 alternative answers of which only one is correct. The number of ways, in which a candidate answers all six questions such that exactly four of the answers are correct, is______
23. If 
then the value of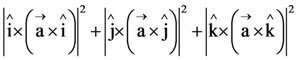 
is equal to ______
24. Let PQ be a diameter of the circle x2 + y2 = 9. If α and β are the lengths of the perpendiculars from P and Q on the straight line, x + y = 2 respectively, then the maximum value of αβ is____
25. If the variance of the following frequency distribution :
Class : 10-20 20-0 30-40
Frequency : 2 x 2
is 50, then x is equal to _______
Latest Govt Job & Exam Updates: