JEE Main Online Computer Based Test (CBT) Examination Held on 15-04-2018
PHYSICS
1. In a common emitter configuration with suitable bias, it is given that RL is the load resistance and RBE is small signal dynamic resistance (input side). Then, voltage gain, current gain and power gain are given, respectively, by :
β is current gain, IB, IC and IE are respectively base, collector and emitter currents.
(1) 
(2) 
(3) 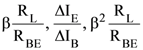
(4) 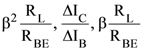
2. A thin uniform tube is bent into a circle of radius r in the vertical plane. Equal volumes of two immiscible liquids, whose densities are ρ1 and ρ2 (ρ1 > ρ2), fill half the circle. The angle θ between the radius vector passing through the common interface and the vertical is :
(1) 
(2) 
(3) 
(4) 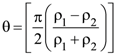
3. 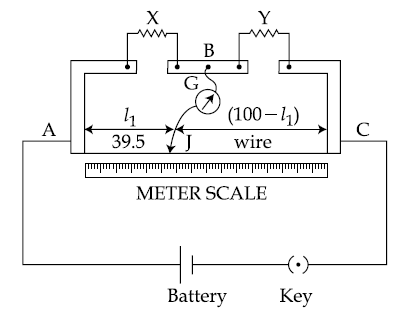
In a meter bridge, as shown in the figure, it is given that resistance Y = 12.5 Ω and that the balance is obtained at a distance 39.5 cm from end A (by Jockey J). After interchanging the resistances X and , a new balance point is found at a distance l2 from end A. What are the values of X and l2?
(1) 19.15 Ω and 39.5 cm
(2) 8.16 Ω and 60.5 cm
(3) 19.15 Ω and 60.5 cm
(4) 8.16 Ω and 39.5 cm
4. An automobile, travelling 40 km/h, can be stopped at a distance of 40 m by applying brakes. If the same automobile is travelling at 80 km/h, the minimum stopping distance, in metres, is (assume no skidding) :
(1) 160 m
(2) 75 m
(3) 150 m
(4) 100 m
5. A given object takes n times more time to slide down a 45° rough inclined plane as it takes to slide down a perfectly smooth 45° The coefficient of kinetic friction between the object and the incline is :
(1) ![]()
(2) 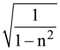
(3) 
(4) 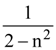
6. A body of mass M and charge q is connected to a spring of spring constant k. It is oscillating along x-direction about its equilibrium position, taken to be at x = 0, with an amplitude A. An electric field E is applied along the x-direction. Which of the following statements is correct?
(1) The new equilibrium position is at a distance ![]()
(2) The total energy of the system is ![]()
(3) The total energy of the system is ![]()
(4) The new equilibrium position is at a distance ![]()
7. The relative error in the determination of the surface area of a sphere is α. Then the relative error in the determination of its volume is :
(1) ![]()
(2) α
(3) ![]()
(4) ![]()
8. A monochromatic beam of light has a frequency ![]() and is propagating along the direction
and is propagating along the direction ![]() It is polarized along the
It is polarized along the![]() direction. The acceptable form for the magnetic field is :
direction. The acceptable form for the magnetic field is :
(1) 
(2) 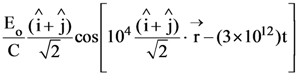
(3) 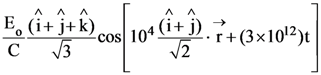
(4) 
9. The energy required to remove the electron from a singly ionized Helium atom is 2.2 times the energy required to remove an electron from Helium atom. The total energy required to ionize the Helium atom completely is :
(1) 109 eV
(2) 34 eV
(3) 79 eV
(4) 20 eV
10. An ideal capacitor of capacitance 0.2 μF is charged to a potential difference of 10 V. The charging battery is then disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5 mH. The current at a time when the potential difference across the capacitor is 5 V, is :
(1) 0.34 A
(2) 0.17 A
(3) 0.25 A
(4) 0.15 A
11. A Carnot’s engine works as a refrigerator between 250 K and 300 K. It receives 500 cal heat from the reservoir at the lower temperature. The amount of work done in each cycle to operate the refrigerator is :
(1) 420 J
(2) 2520 J
(3) 772 J
(4) 2100 J
12. A planoconvex lens becomes an optical system of 28 cm focal length when its plane surface is silvered and illuminated from left to right as shown in Fig-A.
If the same lens is instead silvered on the curved surface and illuminated from other side as in Fig. B, it acts like an optical system of focal length 10 cm. The refractive index of the material of lens is :
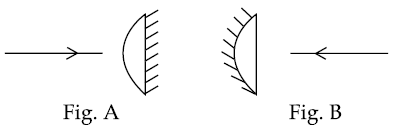
(1) 1.75
(2) 1.50
(3) 1.55
(4) 1.51
13. Two electrons are moving with non-relativistic speeds perpendicular to each other. If corresponding de Broglie wavelengths are λ1 and λ2, their de Broglie wavelength in the frame of reference attached to their centre of mass is :
(1) 
(2) 
(3) λCM = λ1 = λ2
(4) 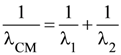
14. Take the mean distance of the moon and the sun from the earth to be 0.4 × 106 km and 150 × 106 km respectively. Their masses are 8 × 1022 kg and 2 × 1030 kg respectively. The radius of the earth is 6400 km. Let ∆F1 be the difference in the forces exerted by the moon at the nearest and farthest points on the earth and ∆F2 be the difference in the force exerted by the sun at the nearest and farthest points on the earth. Then, the number closest to ![]() is :
is :
(1) 10−2
(2) 2
(3) 0.6
(4) 6
15. The equivalent capacitance between A and B in the circuit given below, is :
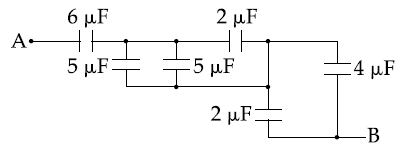
(1) 3.6 μF
(2) 4.9 μF
(3) 5.4 μF
(4) 2.4 μF
16. Light of wavelength 550 nm falls normally on a slit of width 22.0 × 10−5 The angular position of the second minima from the central maximum will be (in radians) :
(1) π/6
(2) π/4
(3) π/8
(4) π/12
17. A tuning fork vibrates with frequency 256 Hz and gives one beat per second with the third normal mode of vibration of an open pipe. What is the length of the pipe ? (Speed of sound in air is 340 ms−1)
(1) 190 cm
(2) 200 cm
(3) 220 cm
(4) 180 cm
18. A body of mass m is moving in a circular orbit of radius R about a planet of mass M. At some instant, it splits into two equal masses. The first mass moves in a circular orbit of radius R/2, and the other mass, in a circular orbit of radius 3R/2. The difference between the final and initial total energies is :
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
19. In a screw gauge, 5 complete rotations of the screw cause it to move a linear distance of 0.25 cm. There are 100 circular scale divisions. The thickness of a wire measured by this screw gauge gives a reading of 4 main scale divisions and 30 circular scale divisions. Assuming negligible zero error, the thickness of the wire is :
(1) 0.3150 cm
(2) 0.4300 cm
(3) 0.2150 cm
(4) 0.0430 cm
20. A solution containing active cobalt ![]() having activity of 0.8 μCi and decay constant λ is injected in an animal’s body. If 1 cm3 of blood is drawn from the animal’s body after 10 hrs of injection, the activity found was 300 decays per minute. What is the volume of blood that is flowing in the body? (1 Ci = 3.7 × 1010 decays per second and at t = 10 hrs e−λt = 0.84)
having activity of 0.8 μCi and decay constant λ is injected in an animal’s body. If 1 cm3 of blood is drawn from the animal’s body after 10 hrs of injection, the activity found was 300 decays per minute. What is the volume of blood that is flowing in the body? (1 Ci = 3.7 × 1010 decays per second and at t = 10 hrs e−λt = 0.84)
(1) 6 liters
(2) 5 liters
(3) 7 liters
(4) 4 liters
21. In the given circuit all resistances are of value R ohm each. The equivalent resistance between A and B is :
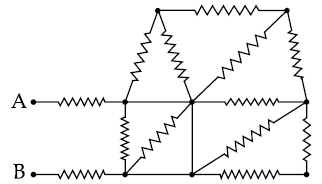
(1) 3R
(2) 2R
(3) 5R/3
(4) 5R/2
22. The velocity-time graphs of a car and a scooter are shown in the figure. (i) The difference between the distance travelled by the car and the scooter in 15 s and (ii) the time at which the car will catch up with the scooter are, respectively.
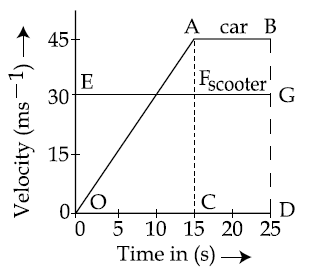
(1) 225.5 m and 10 s
(2) 112.5 m and 15 s
(3) 112.5 and 22.5 s
(4) 337.5 m and 25s
23. 
A uniform rod AB is suspended from a point X, at a variable distance x from A, as shown. To make the rod horizontal, a mass m is suspended from its end A. A set of (m, x) values is recorded. The appropriate variables that give a straight line, when plotted, are :
(1) m, 1/x
(2) m, x2
(3) m, x
(4) m, 1/x2
24. The number of amplitude modulated broadcast stations that can be accommodated in a 300 kHz band width for the highest modulating frequency 15 kHz will be :
(1) 8
(2) 15
(3) 10
(4) 20
25. A charge Q is placed at a distance a/2 above the centre of the square surface of edge as as shown in the figure

The electric flux through the square surface is :
(1) Q/ϵ0
(2) Q/6ϵ0
(3) Q/2ϵ0
(4) Q/3ϵ0
26. A Helmholtz coil has a pair of loops, each with N turns and radius R. They are placed coaxially at distance R and the same current I flows through the loops in the same direction. The magnitude of magnetic field at P, midway between the centres A and C, is given by [Refer to figure given below] :

(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
27. A particle is oscillating on the X-axis with an amplitude 2 cm about the point x0 = 10 cm, with a frequency ω. A concave mirror of focal length 5 cm is placed at the origin (see figure).
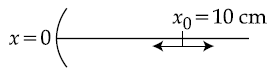
Identify the correct statements.
(A) The image executes periodic motion.
(B) The image executes non-periodic motion.
(C) The turning points of the image are asymmetric w.r.t. the image of the point at x = 10 cm.
(D) The distance between the turning points of the oscillation of the image is ![]()
(1) (B), (D)
(2) (B), (C)
(3) (A), (D)
(4) (A), (C), (D)
28. One mole of an ideal monoatomic gas is compressed isothermally in a rigid vessel to double pressure at room temperature, 27° The work done on the gas will be :
(1) 300 R ln 7
(2) 300 R ln 2
(3) 300 R
(4) 300 R ln 6
29. The B-H curve for a ferromagnet is sown in the figure. The ferromagnet is placed inside a long solenoid with 1000 turns/ cm. The current that should be passed in the solenoid to demagnetize the ferromagnet completely is :
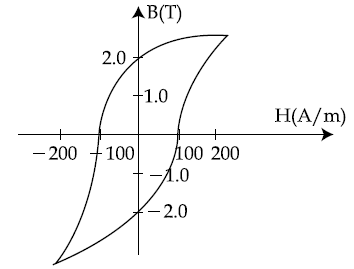
(1) 20 μA
(2) 40 μA
(3) 2 mA
(4) 1 mA
30. A force of 40 N acts on a point B at the end of an L-shaped object, as shown in the figure. The angle θ that will produce maximum moment of the force about point A is given by :
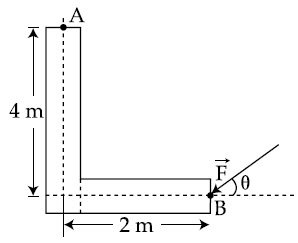
(1) tan θ = 2
(2) tan θ = 4
(3) tan θ = 1/2
(4) tan θ = 1/4
1. Which of the following statements about colloids is False?
(1) When excess of electrolyte is added to colloidal solution, colloidal particle will be precipitated.
(2) Colloidal particles can pass through ordinary filter paper.
(3) When silver nitrate solution is added to potassium iodide solution, a negatively charged colloidal solution is formed.
(4) Freezing pint of colloidal solution is lower than true solution at same concentration of a solute.
2. Ejection of the photoelectron from metal in the photoelectric effect experiment can be stopped by applying 0.5 V when the radiation of 250 nm is used. The work function of the metal is :
(1) 4.5 eV
(2) 5 eV
(3) 5.5 eV
(4) 4 eV
3. In which of the following reactions, an increase in the volume of the container will favour the formation of products?
(1) 2NO2(g) ⇌ 2NO(g) + O2(g)
(2) 4NH3(g) + 5O2(g) ⇌ 4NO(g) + 6H2O(l)
(3) 3O2(g) ⇌ 2O3(g)
(4) H2(g) + I2(g) ⇌ 2HI(g)
4. When an electric current is passed through acidified water, 112 mL of hydrogen gas at N.T.P. was collected at the cathode in 965 seconds. The current passed, in ampere, is :
(1) 0.5
(2) 0.1
(3) 1.0
(4) 2.0
5. ![]()
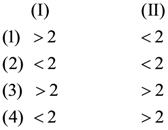
6. The decreasing order of bond angles in BF3, NH3, PF3 and I3− is :
(1) I3− > NH3 > PF3 > BF3
(2) BF3 > I3− > PF3 > NH3
(3) BF3 > NH3 > PF3 > I3−
(4) I3− > BF3 > NH3 > PF3
7. In graphite and diamond, the percentage of p-characters of the hybrid orbitals in hybridization are respectively :
(1) 33 and 25
(2) 33 and 75
(3) 67 and 75
(4) 50 and 75
8. A sample of NaClO3 is converted by heat to NaCl with a loss of 0.16 g of oxygen. The residue is dissolved in water and precipitated as AgCl. The mass of AgCl (in g) obtained will be : (Given : Molar mass of AgCl = 143.5 g mol−1)
(1) 0.54
(2) 0.41
(3) 0.48
(4) 0.35
9. N2O5 decomposes to NO2 and O2 and follows first order kinetics. After 50 minutes, the pressure inside the vessel increases from 50 mmHg to 8.75 mmHg. The pressure of the gaseous mixture after 100 minute at constant temperature will be :
(1) 116.25 mmHg
(2) 106.25 mmHg
(3) 136.25 mmHg
(4) 175.0 mmHg
10. Which of the following arrangements shows the schematic alignment of magnetic moments of antiferromagnetic substance?
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
11. The IUPAC name of the following compound is :

(1) 3-ethyl-4-methylhex-4-ene
(2) 4, 4-dithyl-3-methylbut-2-ene
(3) 4-methyl-3-ethylhex-4-ene
(4) 4-ethyl-3-methylhex-2-ene
12. For which of the following reactions, ∆H is equal to ∆U?
(1) 2HI(g) → H2(g) + I2(g)
(2) 2NO2(g) → N2O4 (g)
(3) N2(g) + 3H2(g) → 2NH3(g)
(4) 2SO2(g) + O2(g) → 2SO3(g)
13. For Na+, Mg2+, F− and O2− ; the correct order of increasing ionic radii is :
(1) Na+ < Mg2+ < F− < O2−
(2) Mg2+ < O2− < Na+ < F−
(3) Mg2+ < Na+ < F− < O2−
(4) O2− < F− < Na+ < Mg2+
14. The minimum volume of water required to dissolve 0.1 g lead (II) chloride to get a saturated solution (Ksp of PbCl2 = 3.2 × 10−8; atomic mass of Pb = 207 u) is :
(1) 17.98 L
(2) 0.18 L
(3) 1.798 L
(4) 0.36 L
15. An ideal gas undergoes a cyclic process a show in Figure.
∆UBC = −5 kJ mol−1, qAB = 2 kJ mol−1
WAB = −5 kJ mol−1, WCA = 3 kJ mol−1
Heat absorbed by the system during process CA is :
(1) −5 kJ mol−1
(2) +5 kJ mol−1
(3) −18 kJ mol−1
(4) 18 kJ mol−1
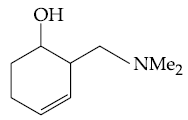
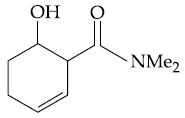
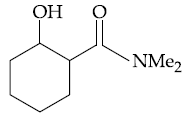
16. The main reduction product of the following compound with NaBH4 in methanol is :
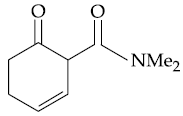
(1) 
(2) 
(3) 
(4) 
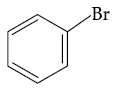
17. Which of the following will most readily give the dehydrohalogenation product?
(1) 
(2) 
(3) 
(4) 
18. The correct combination is :
(1) [NiCl4]2− −square-planar ; [Ni(CN)4] 2− −paramagnetic
(2) [NiCl4] 2− − diamagnetic; [Ni(CO)4] −square-planar
(3) [NiCl4] 2− − tetrahedral; [Ni(CO)4] –paramagnetic
(4) [NiCl4] 2− − paramagnetic; [Ni(CO)4] –tetrahedral
19. Which of the following is a Lewis acid?
(1) B(CH3)3
(2) PH3
(3) NF3
(4) NaH
20. The copolymer formed by addition polymerization of styrene and acrylonitrile in the presence of peroxide is
(1) 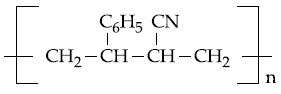
(2) 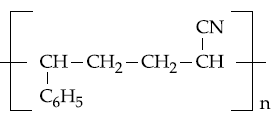
(3) 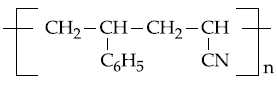
(4) 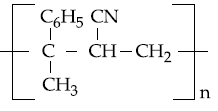
21. The major product of the following reaction is :
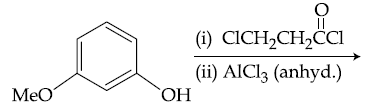
(1) 
(2) 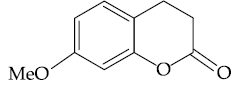
(3) 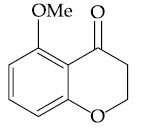
(4) 
22. Xenon hexafluoride on partial hydrolysis produces compounds ‘X’ and ‘Y’ Compounds ‘X’ and ‘Y’ and the oxidation state of Xe are respectively :
(1) XeOF4 (+6) and XeO3 (+6)
(2) XeOF4 (+6) and XeO2F2 (+6)
(3) XeO2F2 (+6) and XeO2 (+4)
(4) XeO2 (+4) and XeO3 (+6)
23. The correct match between times of List-I and List-II is :
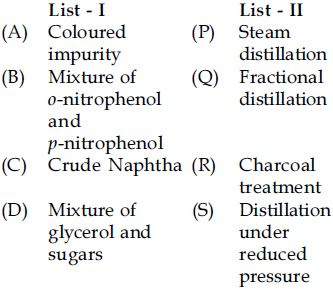
(1) (A)-(R), (B)-(P), (C)-(S), (D)-(Q)
(2) (A)-(R), (B)-(P), (C)-(Q), (D)-(S)
(3) (A)-(P), (B)-(S), (C)-(R), (D)-(Q)
(4) (A)-(R), (B)-(S), (C)-(P), (D)-(Q)
24. A white sodium salt dissolves readily in water to give a solution which is neutral to litmus. When silver nitrate solution is added to the aforementioned solution, a white precipitate is obtained which does not dissolve in dil. nitric acid. The anion is :
(1) SO42−
(2) CO32−
(3) Cl−
(4) S2−
25. In the molecular orbital diagram for the molecular ion, N2+, the number of electrons in the σ2p molecular orbital is :
(1) 2
(2) 1
(3) 0
(4) 3
26. The reagent (s) required for the following conversion are :

(1) (i) B2H6 (ii) SnCl2/HCl (iii) H3O+
(2) (i) B2H6 (ii) DIBAL-H (iii) H3O+
(3) (i) LiAlH4 (ii) H3O+
(4) (i) NaBH4 (ii) Raney Ni/H2 (iii) H3O+
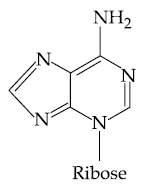
27. Which of the following is the correct structure of Adenosine?
(1) 
(2) 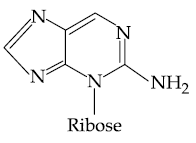
(3) 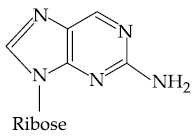
(4) 
28. Identify the pair in which the geometry of the species is T-shape and square-pyramidal, respectively :
(1) IO3− and IO2F2−
(2) XeOF2 and XeOF4
(3) ICl2− and ICl5
(4) ClF3 and IO4−
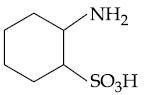
29. Which of the following will not exist in zwitter ionic from at pH = 7?
(1) 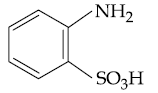
(2) 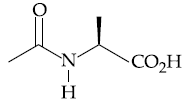
(3) 
(4) 
30. The increasing order of nitration of the following compounds is :

(1) (a) < (b) < (d) < (c)
(2) (b) < (a) < (d) < (c)
(3) (b) < (a) < (c) < (d)
(4) (a) < (b) < (c) < (d)
1. Consider the following two binary relations on the set A = {a, b, c} :
R1 = {(c, a), (b, b), (a, c), (c, c), (b, c), (a, a)} and
R2 = {(a, b), (b, a), (c, c), (c, a), (a, a), (b, b), (a, c)}.
Then :
(1) R1 is not symmetric but it is transitive.
(2) both R1 and R2 are transitive.
(3) both R1 and R2 are not symmetric.
(4) R2 is symmetric but it is not transitive.
2. A box ‘A’ contains 2 white, 3 red and 2 black balls. Another box ‘B’ contains 4 white, 2 red and 3 black balls. If two balls are drawn at random, without replacement, from a randomly selected box and one ball turns out to be white while the other ball turns out to be red, then the probability that both balls are drawn from box ‘B’ is :
(1) 7/16
(2) 7/8
(3) 9/32
(4) 9/16
3. An angle between the plane, x + y + z = 5 and the line of intersection of the planes, 3x + 4y + z – 1 = 0 and 5x + 8y + 2z + 14 = 0, is :
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
4. Two parabolas with a common vertex and with axes long x-axis and y-axis, respectively, intersect each other in the first quadrant. If the length of the latus rectum of each parabola is 3, then the equation of the common tangent to the two parabolas is :
(1) x + 2y + 3 = 0
(2) 4(x + y) + 3 = 0
(3) 3(x + y) + 4 = 0
(4) 8(2x + y) + 3 = 0
5. If x1, x2, . . ., xn and  are two A.P.s such that x3 = h2 = 8 and x8 = h7 = 20, then x5∙h10 equals :
are two A.P.s such that x3 = h2 = 8 and x8 = h7 = 20, then x5∙h10 equals :
(1) 3200
(2) 2560
(3) 2650
(4) 1600
6. If (P ⋀ ~q) ⋀ (p ⋀ r) → ~ p ⋁ q is false, then the truth values of p, q and r are, respectively :
(1) T, F, T
(2) F, F, F
(3) F, T, F
(4) T, T, T
7. Let S be the set of all real values of k for which the system of linear equations
x + y + z = 2
2x + y – z = 3
3x + 2y + kz = 4
has a unique solution. Then S is :
(1) an empty set
(2) equal to R – {0}
(3) equal to R
(4) equal to {0}
8. The area (i9n sq. units) of the region {x ϵ R : x ≥ 0, y ≥ 0, y ≥ x – 2 and y ≤ √x}, is :
(1) 13/3
(2) 10/3
(3) 5/3
(4) 8/3
9. If the tangents drawn to the hyperbola 4y2 = x2 + 1 intersect the co-ordinate axes at the distinct points A and B, then the locus of the mid point of AB is :
(1) x2 – 4y2 + 16x2y2 = 0
(2) 4x2 – y2 – 16x2y2 = 0
(3) 4x2 – y2 + 16x2y2 = 0
(4) x2 – 4y2 – 16x2y2 = 0
10. If β is one of the angles between the normals to the ellipse, x2 + 3y2 = 9 at the points (3 cos θ, √3 sinθ) and (−3 sin θ, √3 cos θ); 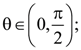 then
then ![]() is equal to :
is equal to :
(1) √3/4
(2) 2/√3
(3) √2
(4) 1/√3
11. If ![]() are unit vectors such that
are unit vectors such that ![]() is equal to :
is equal to :
(1) √15/4
(2) √15/16
(3) 15/16
(4) 1/4
12. n-digit numbers are formed suing only three digits 2, 5 and 7. The smallest value of n for which 900 such distinct numbers can be formed, is :
(1) 9
(2) 6
(3) 7
(4) 8
13. If x2 + y2 + sin y = 4, then the value of ![]() at the point (−2, 0) is :
at the point (−2, 0) is :
(1) −34
(2) −32
(3) 4
(4) −2
14. If tan A and tan B are the roots of the quadratic equation, 3x2 – 10x – 25 = 0, then the value of 3 sin2(A + B) – 10 sin(A + B) ∙ cos(A + B) – 25 cos2(A + B) is :
(1) 25
(2) 10
(3) −25
(4) −10
15. The value of the integral 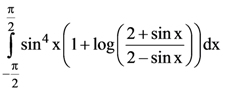 is :
is :
(1) ![]()
(2) 3/4
(3) ![]()
(4) 0
16. The set of all α ϵ R, for which ![]() is a purely imaginary number, for all z ϵ C satisfying |z| = 1 and Re z ≠ 1 is :
is a purely imaginary number, for all z ϵ C satisfying |z| = 1 and Re z ≠ 1 is :
(1) equal to R
(2) an empty set
(3) {0}
(4) ![]()
17. Let y = (x) be the solution of the differential equation ![]() where
where

If y (0) = 0, the ![]() is :
is :
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
18. A variable plane passes through a fixed point (3, 2, 1) and meets x, y and z axes at A, B and C respectively. A plane is drawn parallel to yz-plane through A, a second plane is drawn parallel zx-plane through B and a third plane is drawn parallel to xy-plane through C. Then the locus of the point of intersection of these three planes, is :
(1) ![]()
(2) ![]()
(3) ![]()
(4) x + y + z = 6
19. The mean of a set of 30 observations is 75. If each observation is multiplied by a non-zero number λ and then each of them is decreased by 25, their mean remains the same. Then λ is equal to :
(1) 4/3
(2) 1/3
(3) 2/3
(4) 10/3
20. Let S = {λ, μ) ϵ R × R : f(t) = (|λ| e|t| − μ), sin (2|t|), t ϵ R, is a differentiable function}. Then S is a subset of :
(1) R × (−∞, 0)
(2) R × [0, ∞)
(3) [0, ∞) × R
(4) (−∞, 0) × R
21. If n is the degree of the polynomial, 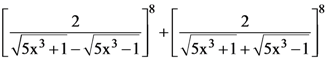 and m is the coefficient of xn in it, then the ordered pair (n, m) is equal to :
and m is the coefficient of xn in it, then the ordered pair (n, m) is equal to :
(1) (12, (20)4)
(2) (8, 5(10)4)
(3) (24, (10)8)
(4) (12, 8(10)4)
22. An aeroplane flying at a constant speed, parallel to the horizontal ground, √3 km above it, is observed at an elevation of 60° from a point on the ground. If, after five seconds, its elevation from the same point, is 30°, then the speed (in km/hr) of the aeroplane, is :
(1) 750
(2) 1440
(3) 1500
(4) 720
23. If b is the first term of an infinite G.P. whose sum is five, then b lies in the interval :
(1) [10, ∞)
(2) (−∞, −10]
(3) (−10, 0)
(4) (0, 10)
24. Let A be a matrix such that  is a scalar matrix and |3A| = 108. Then A2 equals :
is a scalar matrix and |3A| = 108. Then A2 equals :
(1) 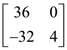
(2) 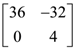
(3) 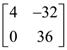
(4) 
25. If a right circular cone, having maximum volume, is inscribed in a sphere of radius 3 cm, then the the curved surface area (in cm2) of this cone is :
(1) 8√3 π
(2) 8√2 π
(3) 6√2 π
(4) 6√3 π
26. If 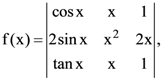 then
then ![]()
(1) does not exist.
(2) exists and is equal to 0.
(3) exists and is equal to 2.
(4) exists and is equal to −2.
27. If λ ϵ R is such that the sum of the cubes of the roots of the equation, x2 + (2 – λ)x + (10 – λ) = 0 is minimum, then the magnitude of the difference of the roots of this equation is :
(1) 4√2
(2) 20
(3) 20√5
(4) 2√7
28. A circle passes through the points (2, 3) and (4, 5). If its centre lies on the line, y – 4x + 3 = 0, then its radius is equal to :
(1) 2
(2) √2
(3) √5
(4) 1
29. In a triangle ABC, coordinates of A are (1, 2) and the equations of the medians through B and C are respectively, x + y = 5 and x = 4. Then area of ∆ABC (in sq. units) is :
(1) 12
(2) 4
(3) 5
(4) 9
30. If ![]() then ∫f(x) dx is equal to :
then ∫f(x) dx is equal to :
(where C is a constant of integration)
(1) 12 loge |1 – x| − 3x + C
(2) −12 loge |1 – x| + 3x + C
(3) −12 loge |1 – x| − 3x + C
(4) 12 loge |1 – x| + 3x + C
Latest Govt Job & Exam Updates: