Mathematics
SECTION – 1 : (One or More Than One Options Correct Type)
This section contains 10 multiple choice questions. Each question has four choices (A), (B), (C) and (D) out of which ONE or MORE THAN ONE are correct.
1. Let f : [a, b] → [1, ∞) be a continuous function and let g : R → R be defined as
(A) g(x) is continuous but not differentiable at a
(B) g (x) is differentiable on R
(C) g(x) is continuous but not differentiable at b
(D) g(x) is continuous and differentiable at either a or b but not both
2. For every pair of continuous functions f, g:[0, 1] → R such that max {f(x) : x ∈ [0, 1]} = max {g(x) : x ∈ [0, 1}, the correct statement(s) is (are)
(A) 
(B) 
(C) 
(D) 
3. Let M be a 2 × 2 symmetric matrix with integer entries. Then M is invertible if
(A) the first column of M is the transpose of the second row of M
(B) the second row of M is the transpose of first column of M
(C) M is a diagonal matrix with nonzero entries in the main diagonal
(D) the product of entries in the main diagonal of M is not the square of an integer
4. Let 
be three vectors each of magnitude √2 and the angle between each pair of them is π/3. If 
is a nonzero vector perpendicular to 
is a nonzero vector perpendicular to 
and 
then
(A) 
(B) 
(C) 
(D) 
5. From a point P(λ, λ, λ), perpendiculars PQ and PR are drawn respectively on the lines y = x = z = 1 and y = −x, z = −1. If P is such that ∠QPR is a right angle, then the possible value(s) of λ is (are)
(A) √2
(B) 1
(C) −1
(D) −√2
6. Let M and N be two 3 × 3 matrices such that MN = NM. Further, if M ≠ N2 and M2 = N4, then
(A) determinant of 
(B) there is a 3 × 3 non-zero matrix U such that 
is the zero matrix
(C) determinant of 
(D) for a 3 × 3 matrix U, if 
equals the zero matrix then U is the zero matrix
7. Let f : (0, ∞) → R be given by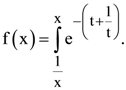 
Then
(A) f(x) is monotonically increasing on [1, ∞)
(B) f(x) is monotonically decreasing on (0, 1)
(C) 
for all x ∈ (0, ∞)
(D) 
is an odd function of x on R
8. Let f: 
be given by f(x) = (log(sec x + tan x))3. Then
(A) f(x) is an odd function
(B) f(x) is a non-one function
(C) f(x) is an onto function
(D) f(x) is an even function
9. A circle S passes through the point (0, 1) and is orthogonal to the circles (x – 1)2 + y2 = 16 and x2 + y2 = 1. Then
(A) radius of S is 8
(B) radius of S is 7
(C) centre of S is (−7, 1)
(D) centre of S is (−8, 1)
10. Let a ∈ R and let f : R → R be given by f(x) = x5 – 5x + a. Then
(A) f(x) has three real roots if a > 4
(B) f(x) has only one real root if a > 4
(C) f(x) has three real roots if a < – 4
(D) f(x) has three real roots if – 4 < a < 4
SECTION – 2 : (One Integer Value Correct Type)
This section contains 10 questions. Each question, when worked out will result in one integer from 0 to 9 (both inclusive).
11. The slope of the tangent to the curve (y – x5)2 = x(1 + x2)2 at the point (1, 3) is
12. Let f : [0, 4π] → [0, π] be defined by f(x) = cos−1 (cos x). The number of points x ∈ [0, 4π] satisfying the equation 
13. The largest value of the non-negative integer a for which
is
14. Let f : R → R and g : R → R be respectively given by f(x) = |x| + 1 and g(x) = x2 + 1. Define h : R → R by 
The number of points at which h(x) is not differentiable is
15. For a point P in the plane, let d1(P) and d2(P) be the distance of the point P from the lines x – y = 0 and x + y = 0 respectively. The area of the region R consisting of all points P lying in the first quadrant of the plane and satisfying 2 ≤ d1(P) + d2(P) ≤ 4, is
16. Let n1 < n2 < n3 < n4 < n5 be positivie integers such that n1 + n2 + n3 + n4 + n5 = 20. Then the number of such distinct arrangements (n1, n2, n3, n4, n5) is
17. The value of 
is
18. Let 
be three non-coplanar unit vectors such that the angle between every pair of them is π/3. If 
where p, q and r are scalars, then the value of 
19. Let a, b, c be positive integers such that b/a is an integer. If a, b, c are in geometric progression and the arithmetic mean of a, b, c is b + 2, then the value of 
is
20. Let n ≤ 2 be an integer. Take n distinct points on a circle and join each pair of points by a line segment. Color the line segment joining every pair of adjacent points by blue and the rest by red. If the number of red and blue line segments are equal, then the value of n is
Latest Govt Job & Exam Updates: