JEE MAIN (AIEEE) Past Exam Paper-2005
Mathematics
1. If C is the mid point of AB and P is any point outside AB, then
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
2. Let P be the point (1, 0) and Q a point on the locus y2 = 8x. The locus of mid point of PQ is
(1) x2 – 4y + 2 = 0
(2) x2 + 4y + 2 = 0
(3) y2 + 4 x + 2 = 0
(4) y2 – 4x + 2 = 0
3. If in a frequency distribution, the Mean and Median are 21 and 22 respectively, then its Mode is approximately
(1) 24.0
(2) 25.5
(3) 20.5
(4) 22.0
4. Let R = {(3, 3), (6, 6), (9, 9), (12, 12), (6, 12), (3, 9), (3, 12), (3, 6)} be a relation on the set
(1) reflexive and symmetric only
(2) an equivalence relation
(3) reflexive only
(4) reflexive and transitive only
5. If A2 – A + I = O, then the inverse of A is
(1) I – A
(2) A – I
(3) A
(4) A + I
6. If the cube roots of unity are 1, ω, ω2, then the roots of the equation (x – 1)3 + 8 = 0 are
(1) −1, 1 + 2ω, 1 + 2ω2
(2) −1, 1−2ω, 1 – 2ω2
(3) −1, −1, −1
(4) −1, −1 + 2ω, −1 − 2ω2
7. 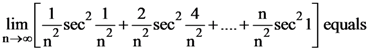
(1) (1/2) tan 1
(2) tan 1
(3) (1/2) cosec 1
(4) (1/2) sec 1
8. Area of the greatest rectangle that can be inscribed in the ellipse 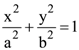 is
is
(1) a/b
(2) √ab
(3) ab
(4) 2ab
9. The differential equation representing the family of curves y2 = 2c(x + √c), where c > 0, is a parameter, is of order and degree as follows
(1) order 2, degree 2
(2) order 1, degree 3
(3) order 1, degree 1
(4) order 1, degree 2
10. ABC is a triangle. Forces ![]() acting along IA, IB and IC respectively are in equilibrium, where I is the incentre of ∆ABC. Then
acting along IA, IB and IC respectively are in equilibrium, where I is the incentre of ∆ABC. Then ![]() is
is
(1) cos A : cos B : cos C
(2) ![]()
(3) ![]()
(4) sin A : sin B : sin C
11. If the coefficients of r th, (r + 1) th and (r + 2)th terms in the binomial expansion (1 + y)m are in AP, then m and r satisfy the equation
(1) m2 – m(4r – 1) + 4r2 + 2 = 0
(2) m2 – m(4r + 1) + 4r2 – 2 = 0
(3) m2 – m(4r + 1) + 4r2 + 2 = 0
(4) m2 – m(4r – 1) + 4r2 – 2 = 0
12. In a triangle PQR, ∠R = π/2. If tan(P/2) and tan(Q/2) are the roots of ax2 + bx + c = 0, a ≠0, then
(1) b = a + c
(2) b = c
(3) c = a + b
(4) a = b + c
13. If the letters of the word SACHIN are arranged in all possible ways and these words are written out as in dictionary, then the word SACHIN appears at serial number
(1) 602
(2) 603
(3) 600
(4) 601
14. The value of 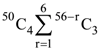 is
is
(1) 56C4
(2) 56C3
(3) 55C3
(4) 55C4
15. If 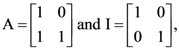 then which one of the following holds for all n ≥ 1, by the principle of mathematical induction?
then which one of the following holds for all n ≥ 1, by the principle of mathematical induction?
(1) An = 2n−1 A + (n – 1)I
(2) An = n A + (n – 1)I
(3) An = 2n−1 A – (n – 1) I
(4) An = n A – (n – 1)I
16. If the coefficient of x7 in 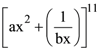 equals the coefficients of x−7 in
equals the coefficients of x−7 in 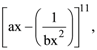 then a and b satisfy the relation
then a and b satisfy the relation
(1) ab = 1
(2) a/b = 1
(3) a + b = 1
(4) a – b = 1
17. Let f : (−1, 1) → B, be a function defined by 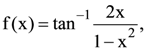 then f is both one-one and onto when B is the interval
then f is both one-one and onto when B is the interval
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
18. If z1 and z2 are two non-zero complex numbers such that |z1 + z2| = |z1| + |z2|, then
(1) −π/2
(2) 0
(3) −π
(4) π/2
19. If 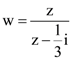 and |w| = 1, then z lies on
and |w| = 1, then z lies on
(1) a parabola
(2) a straight line
(3) a circle
(4) an ellipse
20. If a2 + b2 + c2 = −2 and 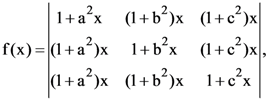 then f(x) is a polynomial of degree
then f(x) is a polynomial of degree
(1) 2
(2) 3
(3) 0
(4) 1
21. The system of equations
α x + y + z = α – 1
x + α y + z = α – 1
x + y + α z = α – 1
has no solution, if α is
(1) 1
(2) not −2
(3) either −2 or 1
(4) −2
22. The value of a for which the sum of the squares of the roots of the equation x2 – (a – 2)x – a – 1 = 0 assume the least value is
(1) 2
(2) 3
(3) 0
(4) 1
23. If the roots of the equation x2 – bx + c = 0 be two consecutive integers, then b2 – 4c equals
(1) 1
(2) 2
(3) 3
(4) −2
24. Suppose f(x) is differentiable at x = 1 and ![]() then f’(1) equals
then f’(1) equals
(1) 6
(2) 5
(3) 4
(4) 3
25. Let f be differentiable for all x. if f(1) = −2 and f'(x) ≥ 2 for x ∈ [1, 6], then
(1) f(6) = 5
(2) f(6) < 5
(3) f(6) < 8
(4) f(6) ≥ 8
26. If f is a real-valued differentiable function satisfying |f(x) – f(y)| ≤ (x – y)2, x, y ∈ R and f(0) = 0 then f(1) equals
(1) 1
(2) 2
(3) 0
(4) −1
27. If x is so small that x3 and higher powers of x may be neglected, then 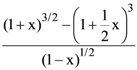 may be approximated as
may be approximated as
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
28. If 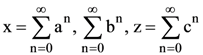 where a, b, c are in AP and |a| < 1, |b| < 1, |c| < 1, then x, y z are in
where a, b, c are in AP and |a| < 1, |b| < 1, |c| < 1, then x, y z are in
(1) HP
(2) AGP
(3) AP
(4) GP
29. In a triangle ABC, let ∠C = π/2, if r is the inradius and R is the circumradius of the triangle ABC, then 2(r + R) equals
(1) c + a
(2) a + b + c
(3) a + b
(4) b + c
30. If cos−1 x – cos−1 (y/2) = α, then 4x2 – 4xy cos α + y2 is equal to
(1) − 4 sin2 α
(2) 4 sin2 α
(3) 4
(4) 2 sin 2α
31. If in a ∆ABC, the altitudes from the vertices A, B, C on opposite sides are in HP, then sin A, sin B, sin C are in
(1) HP
(2) AGP
(3) AP
(4) GP
32. The normal to the curve x = a(cos θ + θ sin θ), y = a (sin θ – θ cos θ) at any point ‘θ’ is such that
(1) it is at a constant distance from the origin
(2) it passes through (a π/2, −a)
(3) it makes angle π/2 + θ with the x-axis
(4) both a and c
33. A function is matched below against an interval where it is supposed to be increasing. Which of the following pairs is incorrectly matched?
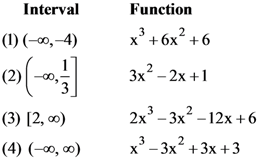
34. Let α and β be the distinct roots of ax2 + bx + c = 0, then
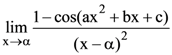
(1) ![]()
(2) 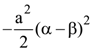
(3) 0
(4) 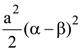
35. If ![]() then the solution of the equation is
then the solution of the equation is
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
36. The line parallel to the x-axis and passing through the intersection of the lines ax + 2by + 3b = 0 and bx – 2ay – 3a = 0, where (a, b) ≠ (0, 0) is
(1) above the x-axis at a distance of (2/3) from it
(2) above the x-axis at a distance of (3/2) from it
(3) below the x-axis at a distance of (2/3) from it
(4) below the x-axis at a distance of (3/2) from it
37. A spherical iron ball 10 cm in radius is coated with a layer of ice of uniform thickness that melts at a rate of 50 cm3/min. when the thickness of ice is 15 cm, then the rate at which thickness of ice decreases, is
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
38. 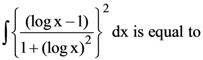
(1) 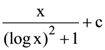
(2) 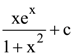
(3) 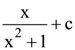
(4) 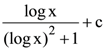
39. Let f:R →R be a differentiable function having f(2) = 6, f'(2) = (1/48).
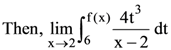
(1) 18
(2) 12
(3) 36
(4) 24
40. Let f(x) be a non-negative continuous function such that the area bounded by the curve y = f(x), x-axis and the ordinates x = π/4 and x = β > π/4 is ![]() Then f(π/2) is
Then f(π/2) is
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
41. If 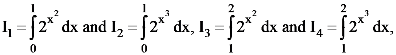 then
then
(1) I3 > I4
(2) I3 = I4
(3) I1 > I2
(4) I2 > I1
42. The area enclosed between the curve y = loge(x + e) and the coordinate axes is
(1) 4
(2) 3
(3) 2
(4) 1
43. The parabolas y2 = 4x and x2 = 4y divide the square region bounded by the lines x = 4, y = 4 and the coordinate axes. If S1, S2, S3 are respectively the areas of these parts numbered from top to bottom, then S1 : S2 : S3 is
(1) 1 : 1 : 1
(2) 2 : 1 : 2
(3) 1 : 2 : 3
(4) 1 : 2 : 1
44. If the plane 2ax – 3ay + 4az + 6 = 0 passes through the mid point of the line joining the centres of the spheres x2 + y2 + z2 + 6x – 8y – 2z = 13 and x2 + y2 + z2 – 10x + 4y – 2z = 8, then a equals
(1) 2
(2) −2
(3) 1
(4) −1
45. The distance between the line ![]() and the plane
and the plane ![]() is
is
(1) 10/3
(2) 3/10
(3) 10/3√3
(4) 10/9
46. For any vector ![]() the value of
the value of ![]() is equal to
is equal to
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
47. If non-zero numbers a, b, c are in HP, then the straight line ![]() always passes through a fixed point. That point is
always passes through a fixed point. That point is
(1) (1, −1/2)
(2) (1, −2)
(3) (−1, −2)
(4) (−1, 2)
48. If a vertex of a triangle is (1, 1) and the mid points of two sides through this vertex are (−1, 2) and (3, 2), then the centroid of the triangle is,
(1) (1/3, 7/3)
(2) (1, 7/3)
(3) (−1/3, 7/3)
(4) (−1, 7/3)
49. If the circles x2 + y2 + 2ax + cy + a = 0 and x2 + y2 – 3ax + dy – 1 = 0 intersect in two distinct points P and Q, then the line 5x + by – a = 0 passes through P and Q for
(1) exactly two values of a
(2) infinitely many values of a
(3) no value of a
(4) exactly one value of a
50. A circle touches the x-axes and also touches the circle with centre at (0, 3) and radius 2. The locus of the centre of the circle is
(1) a parabola
(2) a hyperbola
(3) a circle
(4) an ellipse
51. If a circle passes through the point (a, b) and cuts the circle x2 + y2 = p2 orthogonally, then the equation of the locus of its centre is
(1) 2ax + 2by – (a2 + b2 + p2) = 0
(2) x2 + y2 – 2ax – 3by + (a2 – b2 – p2) = 0
(3) 2ax + 2by – (a2 – b2 + p2) =0
(4) x2 + y2 – 3ax – 4by + (a2 + b2 – p2) = 0
52. An ellipse has OB as semi minor axis, F and F’ its foci and the angle FBF’ is a right angle. Then, the eccentricity of the ellipse is
(1) 1/√3
(2) 1/4
(3) 1/2
(4) 1/√2
53. The locus of a point P(α, β) moving under the condition that the line y =α x + β is a tangent to the hyperbola 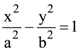 is
is
(1) a hyperbola
(2) a parabola
(3) a circle
(4) an ellipse
54. If the angel θ between the line ![]() and the plane 2x – y + √λz + 4 = 0 is such that sin θ = 1/3. The value of λ is
and the plane 2x – y + √λz + 4 = 0 is such that sin θ = 1/3. The value of λ is
(1) −4/3
(2) 3/4
(3) −3/5
(4) 5/3
55. The angle between the lines 2x = 3y = −z and 6x = −y = −4z is
(1) 30°
(2) 45°
(3) 90°
(4) 0°
56. Let A and B be two events such that ![]() P(A ∩ B) = 1/4 , and P(Ā) = 1/4, where Ā stands for complement of event A. Then, events A and B are
P(A ∩ B) = 1/4 , and P(Ā) = 1/4, where Ā stands for complement of event A. Then, events A and B are
(1) mutually exclusive and independent
(2) independent but not equally likely
(3) equally likely but not independent
(4) equally likely and mutually exclusive
57. Three houses are available in a locality. Three persons apply for the houses. Each applies for one house without consulting others. The probability that all the three apply for the same house, is
(1) 7/9
(2) 8/9
(3) 1/9
(4) 2/9
58. A random variable X has poisson distribution with mean 2. Then, P(X > 1.5) equals
(1) 3/e2
(2) 1 – (3/e2)
(3) 0
(4) 2/e2
59. Two points A and B move from rest along a straight line with constant acceleration f and f’ respectively. If A takes m second more than B and describes ‘n’ unit more than B in acquiring the same speed, then
(1) (f’ – f)n = (1/2) ff’ m2
(2) 1/2 (f + f’)m = ff’ n2
(3) (f + f’)m2 = ff’ n
(4) (f − f’)m2 = ff’ n
60. A lizard, at an initial distance of 21 cm behind an insect, moves from rest with an acceleration of 2 cm/s2 and pursues the insect which is crawling uniformly along a straight line at a speed of 20 cm/s. Then, the lizard will catch the insect after
(1) 24 s
(2) 21 s
(3) 1 s
(4) 20 s
61. The resultant R of two forces acting on a particle is at right angles to one of them and its magnitude is one third of the other force. The ratio of larger force to smaller one is
(1) 3 : 2√2
(2) 3: 2
(3) 3 : √2
(4) 2 : 1
62. Let ![]()
![]() Then,
Then, ![]() depends on
depends on
(1) neither x nor y
(2) both x and y
(3) only x
(4) only y
63. Let a, b and c be distinct non-negative numbers. If the vectors ![]() lie in a plane, then c is
lie in a plane, then c is
(1) the harmonic mean of a and b
(2) equal to zero
(3) the arithmetic mean of a and b
(4) the geometric mean of a and b
64. If ![]() are non-coplanar vectors and λ is a real number, then
are non-coplanar vectors and λ is a real number, then ![]() for
for
(1) exactly two values of λ
(2) exactly three values of λ
(3) no value of λ
(4) exactly one value of λ
65. A and B are two like parallel forces. A couple of moment H lies in the plane of A and B and is contained with them. The resultant of A and B after combining is displaced through a distance
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
66. The sum of the series ![]()
(1) ![]()
(2) ![]()
(3) ![]()
(4) ![]()
67. Let x1, x2 ………., xn be n observations such that ![]() Then, a possible value of n among the following is
Then, a possible value of n among the following is
(1) 12
(2) 9
(3) 18
(4) 15
68. A particle is projected from a point O with velocity u at an angle of 60° with the horizontal. When it is moving in a direction at right angle to its direction at O, then its velocity is given by
(1) u/√3
(2) 2u/3
(3) u/2
(4) u/3
69. If both the roots of the quadratic equation x2– 2kx + k2 + k – 5 = 0 are less than 5, then k lies in the interval
(1) [4, 5]
(2) (−∞, 4)
(3) (6, ∞)
(4) (5, 6]
70. If a1, a2, a3, …………., an,… are GP, then the determinant  is equal to
is equal to
(1) 2
(2) 4
(3) 0
(4) 1
71. A real valued function f(x) satisfied the functional equation
f(x – y) = f(x) f(y) – f(a – x) f(a + y)
where a is a given constant and f(0) = 1, f(2a – x) is equal to
(1) f(−x)
(2) f(a) + f(a – x)
(3) f(x)
(4) −f(x)
72. If the equation anxn + an−1xn−1 + ……+a1x = 0
a1 ≠ 0, n ≥ 2, has a positive roots x = α, then the equation
nanxn−1 + (n – 1)an−1xn−2 + …+a1 = 0 has a positive root, which is
(1) equal to α
(2) greater than or equal to α
(3) smaller than α
(4) greater than α
73. The value of 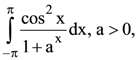 is
is
(1) 2π
(2) π/a
(3) π/2
(4) aπ
74. The plane x + 2y – z = 4 cuts the sphere x2 + y2 + z2 – x + z – 2 = 0 in a circle of radius
(1) √2
(2) 2
(3) 1
(4) 3
75. If the pair of lines ax2 + 2(a + b)xy + by2 = 0 lie along diameters of a circle and divide the circle into four sectors such that the area of one of the sectors is thrice the area of another sector, then
(1) 3a2 + 2ab + 3b2 = 0
(2) 3a2 + 10ab + 3b2 = 0
(3) 3a2 − 2ab + 3b2 = 0
(4) 3a2 – 10ab + 3b2 = 0
Latest Govt Job & Exam Updates: