Physics
Section-A
1. The decay of a proton to neutron is:
(a) Not possible as proton mass is less than the neutron mass
(b) Always possible as it is associated only with β+ decay
(c) Possible only inside the nucleus
(d) Not possible but neutron to proton conversion is possible
2. An object of mass m1 collides with another object of mass m2, which is at rest. After the collision, the objects move at equal speeds in opposite directions. The ratio of the masses m2: m1 is:
(a) 2 : 1
(b) 1 : 1
(c) 1 : 2
(d) 3 : 1
3. A plane electromagnetic wave propagating along y-direction can have the following pair of electric field 
and magnetic field 
components.
(a) Ex, Bz or Ez, Bx
(b) Ey, Bx or Ex, By
(c) Ex, By or Ey, Bx
(d) Ey, By or Ez, Bz
4. A solid cylinder of mass m is wrapped with an inextensible light string and is placed on a rough inclined plane as shown in the figure. The frictional force acting between the cylinder and the inclined plane is :
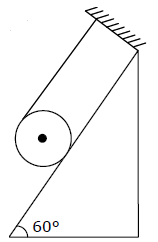
[The coefficient of static friction, μs, is 0.4]
(a) 
(b) 0
(c) mg/5
(d) 5 mg
5. An ideal gas in a cylinder is separated by a piston in such a way that the entropy of one part is S1 and that of the other part is S2. Given that S1 > S2. If the piston is removed then the total entropy of the system will be:
(a) S1 + S2
(b) S1 – S2
(c) S1 × S2
(d) S1/S2
6. The time taken for the magnetic energy to reach 25% of its maximum value, when a solenoid of resistance R, inductance L is connected to a battery, is :
(a) 
(b) 
(c) Infinite
(d) 
7. For an adiabatic expansion of an ideal gas, the fractional change in its pressure is equal to (where λ is the ratio of specific heats) :
(a) 
(b) 
(c) 
(d) 
8. The correct relation between α (ratio of collector current to emitter current) and β (ratio of collector current to base current) of a transistor is :
(a) 
(b) 
(c) 
(d) 
9. In a series LCR circuit, the inductive reactance (XL) is 10 Ω and the capacitive reactance (XC) is 4 Ω. The resistance (R) in the circuit is 6 Ω. Find the power factor of the circuit.
(a) 1/√2
(b) √3/2
(c) 1/2
(d) 1/2√2
10. A proton and an α-particle, having kinetic energies Kp and Kα respectively, enter into a magnetic field at right angles. The ratio of the radii of the trajectory of proton to that α-particle is 2: 1. The ratio of KP: Kα is :
(a) 1 : 8
(b) 1 : 4
(c) 8 : 1
(d) 4 : 1
11. The function of time representing a simple harmonic motion with a period of π/ω is :
(a) cos(ωt) + cos(2ωt) + cos(3ωt)
(b) 
(c) sin2 (ωt)
(d) sin (ωt) + cos (ωt)
12. Consider a uniform wire of mass M and length L. It is bent into a semicircle. Its moment of inertia about a line perpendicular to the plane of the wire passing through the centre is :
(a) 
(b) 
(c) 
(d) 
13. The angular momentum of a planet of mass M moving around the sun in an elliptical orbit is 
The magnitude of the areal velocity of the planet is :
(a) L/M
(b) 2L/M
(c) L/2M
(d) 4L/M
14. The velocity – displacement graph of a particle is shown in the figure.
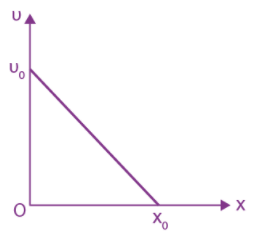
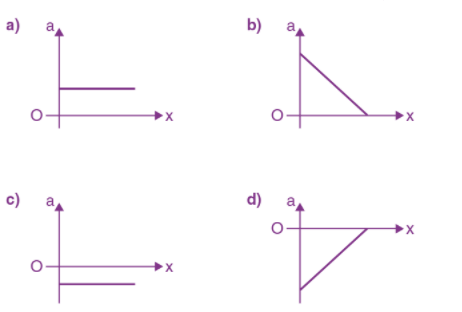
The acceleration – displacement graph of the same particle is represented by:
15. Three rays of light, namely red (R), green (G) and blue (B) are incident on the face PQ of a right angled prism PQR as shown in the figure.
The refractive indices of the material of the prism for red, green and blue wavelengths are 1.27, 1.42 and 1.49 respectively. The colour of the ray(s) emerging out of the face PR is:
(a) Blue
(b) Green
(c) Red
(d) Blue and Green
16. Consider a sample of oxygen behaving like an ideal gas. At 300 K, the ratio of root mean square (rms) velocity to the average velocity of gas molecule would be : (Molecular weight of oxygen is 32 g/mol; R=8.3 JK−1 mol−1)
(a) 
(b) 
(c) 
(d) 
17. A particle of mass m moves in a circular orbit under the central potential field, U(r) = −C/r, where C is a positive constant. The correct radius – velocity graph of the particle’s motion is :
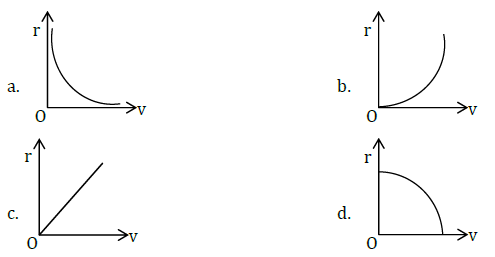
18. Which of the following statements are correct ?
(A) Electric monopoles do not exist whereas magnetic monopoles exist.
(B) Magnetic field lines due to a solenoid at its ends and outside cannot be completely straight and confined.
(C) Magnetic field lines are completely confined within a toroid.
(D) Magnetic field lines inside a bar magnet are not parallel.
(E) χ = —1 is the condition for a perfect diamagnetic material, where χ is its magnetic susceptibility.
Choose the correct answer from the options given below :
(a) (B) and (C) only
(b) (B) and (D) only
(c) (C) and (E) only
(d) (A) and (B) only
19. The speed of electrons in a scanning electron microscope is 1×107 ms−1. If the protons having the same speed are used instead of electrons, then the resolving power of scanning proton microscope will be changed by a factor of:
(a) 1/√1837
(b) √1837
(c) 1837
(d) 1/1837
20. If the angular velocity of earth’s spin is increased such that the bodies at the equator start floating, the duration of the day would be approximately :
[Take g = 10 ms−2, the radius of earth, R = 6400 × 103 m, Take π = 3.14]
(a) 60 minutes
(b) does not change
(c) 84 minutes
(d) 1200 minutes
Section-B
21. Two wires of same length and thickness having specific resistances 6 Ω cm and 3 Ω cm respectively are connected in parallel. The effective resistivity is ρ Ω cm. The value of ρ, to the nearest integer, is _________.
22. A ball of mass 4 kg, moving with a velocity of 10 ms−1, collides with a spring of length 8 m and force constant 100 Nm−1. The length of the compressed spring is x m. The value of x, to the nearest integer, is _______
23. Consider a 72 cm long wire AB as shown in the figure. The galvanometer jockey is placed at P on AB at a distance x cm from A. The galvanometer shows zero deflection.
The value of x, to the nearest integer, is ___________
24. Consider a water tank as shown in the figure. It’s cross-sectional area is 0.4 m2. The tank has an opening B near the bottom whose cross-sectional area is 1 cm2. A load of 24 kg is applied on the water at the top when the height of the water level is 40 cm above the bottom, the velocity of water coming out the opening B is v ms−1. The value of v, to the nearest integer, is …………
[Take value of g to be 10 ms−2]
25. The typical output characteristics curve for a transistor working in the common emitter configuration is shown in the figure.
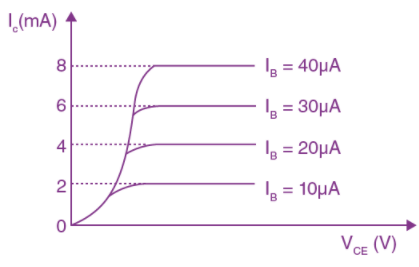
The estimated current gain from the figure is …………..
26. The radius of a sphere is measured to be (7.50 ± 0.85) cm. Suppose the percentage error in its volume is x. The value of x, to the nearest integer x, is …………
27. The projectile motion of a particle of mass 5 g is shown in the figure.
The initial velocity of the particle is 5√2 ms−1 and the air resistance is assumed to be negligible. The magnitude of the change in momentum between the points A and B is X × 102 kgms−1. The value of X, to the nearest integer, is …………………..
28. An infinite number of point charges, each carrying 1 μC charge, are placed along the y-axis at y = 1m, 2m, 4m, 8m ………… The total force on a 1 C point charge, placed at the origin, is X × 103 The value of X, to the nearest integer, is ………
29. A TV transmission tower antenna is at a height of 20 m. Suppose that the receiving antenna is at.
(i) Ground level
(ii) a height of 5 m
The increase in antenna range in case (ii) relative to case (i) is n%. The value of n, to the nearest integer, is
30. A galaxy is moving away from the earth at a speed of 286 km/s. The shift in the wavelength of a redline at 630 nm is X × 10−10 The value of X, to the nearest integer, is [Take the value of speed of light c, as 3 × 108 ms−1]
Chemistry
Section-A
1. Consider the below-given reaction, the product ‘X’ and ‘Y’ respectively are:
2. The charges on the colloidal CdS sol. and TiO2 are, respectively
(a) positive and negative
(b) negative and negative
(c) negative and positive
(d) positive and positive
3. The oxide that shows a magnetic property is:
(a) SiO2
(b) Na2O
(c) Mn3O4
(d) MgO
4. Given below are two statements:
Statement I: Bohr’s theory accounts for the stability and line spectrum of Li+ ion.
Statement II: Bohr’s theory was unable to explain the splitting of spectral lines in the presence of a magnetic field.
In the light of the above statements, choose the most appropriate answer from the options given below:
(a) Both statement I and statement II are true
(b) Statement I is true but statement II is false
(c) Statement I is false but statement II is true
(d) Both statement I and statement II are false
5. Match List-I with List-II:
List-I List-II
(a) Mercury (i) Vapour phase refining
(b) Copper (ii) Distillation Refining
(c) Silicon (iii) Electrolytic Refining
(d) Nickel (iv) Zone Refining
Choose the most appropriate answer from the option given below:
(a) (a)-(ii), (b)-(iii), (c)-(i), (d)-(iv)
(b) (a)-(ii), (b)-(iv), (c)-(iii),(d)-(ii)
(c) (a)-(i), (b)-(iv), (c)-(ii), (d)-(iii)
(d) (a)-(ii), (b)-(iii), (c)-(iv), (d)-(i)
6. Match List-I with List-II:
List-I List-II
(Class of Chemicals) (Example)
(a) Antifertility drug (i) Meprobamate
(b) Antibiotic (ii) Alitame
(c) Tranquilizer (iii) Norethindrone
(d) Artificial Sweetener (iv) Salvarsan
Choose the most appropriate answer from the option given below:
(a) (a)-(iv), (b)-(iii), (c)-(ii), (d)-(i)
(b) (a)-(ii), (b)-(iii), (c)-(iv), (d)-(i)
(c) (a)-(ii), (b)-(iv), (c)-(i), (d)-(iii)
(d) (a)-(iii), (b)-(iv), (c)-(i), (d)-(ii)
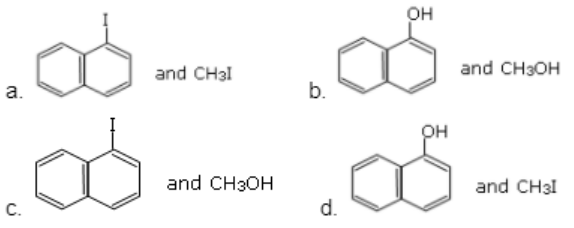
7. Main Products formed during a reaction of 1-methoxy naphthalene with hydroiodic acid are:
8. Consider the given reaction, percentage yield of:
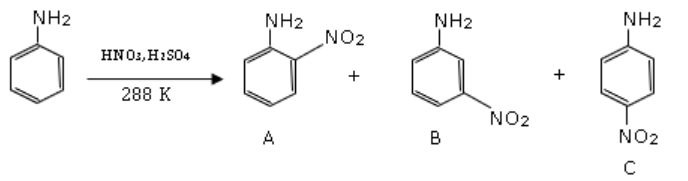
(a) A > C > B
(b) B > C > A
(c) C > B > A
(d) C > A > B
9. An organic compound “A” on treatment with benzene sulphonyl chloride gives compound B. B is soluble in dil. NaOH solution. Compound A is:
(a) C6H5–N–(CH3)2
(b) C6H5–NHCH2CH3
(c) 
(d) C6H5−CH2NHCH3
10. The first ionization energy of magnesium is smaller as a compound to that of elements X and Y but higher than that of Z. The elements X, Y and Z, respectively are:
(a) argon, lithium and sodium
(b) chlorine, lithium and sodium
(c) neon, sodium and chlorine
(d) argon, chlorine and sodium
11. In the following molecule:
The hybridisation of Carbon a, b and c respectively are:
(a) sp3, sp2, sp2
(b) sp3, sp2, sp
(c) sp3, sp, sp
(d) sp3, sp, sp2
12. In the reaction of hypobromite with amide, the carbonyl carbon is lost as:
(a) HCO3−
(b) CO32−
(c) CO2
(d) CO
13. The oxidation states of nitrogen in NO, NO2, N2O and NO3– are in the order of
(a) NO2 > NO3– > NO > N2O
(b) N2O > NO2 > NO > NO3–
(c) NO3– > NO2 > NO > N2O
(d) NO > NO2 > NO3– > N2O
14. Match List-I and List-II:
List-I List-II
(a) Be (i) treatment of cancer
(b) Mg (ii) extraction of metals
(c) Ca (iii) incendiary bombs and signals
(d) Ra (iv) windows of X-ray tubes
(v) bearings for motor engines
Choose the most appropriate answer from the option given below:
(a) (a)-(iii), (b)-(iv), (c)-(ii), (d)-(v)
(b) (a)-(iv), (b)-(iii), (c)-(i), (d)-(ii)
(c) (a)-(iv), (b)-(iii), (c)-(ii), (d)-(i)
(d) (a)-(iii), (b)-(iv), (c)-(v), (d)-(ii)
15. Deficiency of vitamin K causes:
(a) Cheilosis
(b) Increase in blood clotting time
(c) Increase in the fragility of RBCs
(d) Decrease in blood clotting time
16. Given below are two statements:
Statement I: C2H5OH and AgCN both can generate nucleophiles.
Statement II: KCN and AgCN both will generate nitrile nucleophiles with all reaction conditions.
Choose the most appropriate option:
(a) Statement I is false but statement II is true
(b) Statement I is true but statement II is false
(c) Both statement I and statement II are false
(d) Both statement I and statement II are true
17. Given below are two statements:
Statement I: Non-biodegradable wastes are generated by thermal power plants.
Statement II: Biodegradable detergents lead to eutrophication.
In the light of the above statements, choose the most appropriate answer from the options given below.
(a) Statement I is false but statement II is true
(b) Statement I is true but statement II is false
(c) Both statement I and statement II are false
(d) Both statement I and statement II are true
18. A hard substance melts at high temperature and is an insulator in both solid and in molten state. This solid is most likely to be a/an:
(a) Metallic solid
(b) Covalent solid
(c) Ionic solid
(d) Molecular solid
19. The secondary valency and the number of hydrogen bonded water molecule(s) in CuSO4.5H2O, respectively, are:
(a) 6 and 4
(b) 4 and 1
(c) 5 and 1
(d) 6 and 5
20.In a basic medium, H2O2 exhibits which of the following reactions?
(A) Mn2+ → Mn4+
(B) I2 → I−
(C) PbS → PbSO4
(a) (A), (C) only
(b) (A) only
(c) (B) only
(d) (A), (B) only
Section-B
21. The solubility of CdSO4 in water is 8.0 × 10–4 mol L–1. Its solubility in 0.01 M H2SO4 solution is ______ × 10–6 mol L–1. (Round off to the Nearest Integer).
(Assume that solubility is much less than 0.01 M)
22. The molar conductivities at infinite dilution of barium chloride, sulphuric acid and hydrochloric acid are 280, 860 and 426 S cm2 mol–1 The molar conductivity at infinite dilution of barium sulphate is _____ S cm2 mol–1. (Round off to the Nearest Integer).
23. A reaction has a half life of 1 min. The time required for 99.9% completion of the reaction is _____ min. (Round off to the nearest integer) [ Use ln2 = 0.69, ln10 = 2.3]
24. The equilibrium constant KC for this reaction is ______ × 10–2. (Round off to the Nearest Integer).
[Use : R = 8.3 J mol–1 K–1, ln 10 = 2.3
log102 = 0.30, 1 atm = 1 bar]
[antilog (– 0.3) = 0.501]
25. Consider the below reaction where 6.1 g of benzoic acid is used to get 7.8 g of m-bromo benzoic acid.
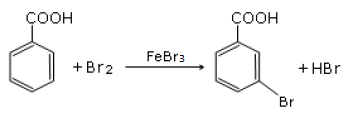
The percentage yield of the product is _____
(Round off to the nearest integer)
[Given : Atomic masses : C : 12.0 u, H : 1.0 u, O : 16.0 u, Br : 80.0 u]
26. A solute A dimerizes in water. The boiling point of a 2 molal solution of A is 100.52ºC. The percentage association of A is ______. (Round off to the nearest integer.)
[Use : Kb for water = 0.52 K kg mol–1]
Boiling point of water = 100ºC]
27. The number of species below that has two lone pairs of electrons in their central atom is ______. (Round off to the Nearest Integer.)
SF4, BF4–, CIF3, AsF3, PCl5, BrF5, XeF4, SF6
28. 10.0 mL of Na2CO3 solution is titrated against 0.2 M HCl solution. The following litre values were obtained in 5 readings 4.8 mL, 4.9 mL, 5.0 mL, 5.0 mL and 5.0 mL. Based on these readings and the convention of titrimetric estimation the concentration of Na2CO3 solution is ____mM
29. In Tollen’s test for aldehyde, the overall number of electron(s) transferred to the Tollen’s reagent formula [Ag(NH3)2]+ per aldehyde group to form silver mirror is ________. (Round off to the Nearest Integer)
30. A xenon compound ‘A’ upon partial hydrolysis gives XeO2F2. The number of lone pairs of electrons presents in compound A is ______. (Round off to the Nearest Integer).
Mathematics
Section-A
1. Let the system of linear equations
4x + λy + 2z = 0
2x – y + z = 0
μx + 2y + 3z = 0, λ, μ ∈ R
Has a non-trivial solution. Then which of the following is true?
(a) μ = 6, λ ∈ R
(b) λ = 2, μ ∈ R
(c) λ = 3, μ ∈ R
(d) μ = −6, λ ∈ R
2. A pole stands vertically inside a triangular park ABC. Let the angle of elevation of the top of the pole from each corner of the park be π/3. If the radius of the circumcircle of △ABC is 2, then the height of the pole is equal to
(a) 1/√3
(b) √3
(c) 2√3
(d) 2√3/3
3. Let in a series of 2n observations, half of them are equal to a and the remaining half are equal to − Also by adding a constant b in each of these observations, the mean and standard deviation of the new set become 5 and 20, respectively. Then the value of a2 + b2 is equal to:
(a) 250
(b) 925
(c) 650
(d) 425
4. Let 
where f is continuous function in [0, 3] such that 
for all t ∈ [0, 1] and 
for all t ∈ {1, 3]. The largest possible interval in which g(3) lies is:
(a) [1, 3]
(b) [−1, −1/2]
(c) [−3/2, −1]
(d) [1/3, 2]
5. If 15 sin4 θ + 10 cos4 θ = 6, for some θ ∈ R, then the value of 27 sec6 θ + 8 cosec6 θ is equal to:
(a) 250
(b) 500
(c) 400
(d) 350
6. Let f : R − {3} → R − {1] be defined by 
Let g : R − R be given as g (x) = 2x − Then, the sum of all the values of x for which f−1 (x) + g−1 (x) = 13/2 is equal to
(a) 7
(b) 5
(c) 2
(d) 3
7. Let S1 be the sum of the first 2n terms of an arithmetic progression. Let S2 be the sum of the first 4n terms of the same arithmetic progression. If (S2 − S1) is 1000, then the sum of the first 6n terms of the arithmetic progression is equal to :
(a) 3000
(b) 7000
(c) 5000
(d) 1000
8. Let S1 = x2 + y2 = 9 and S2 = (x − 2)2 + y2 = 1. Then the locus of the centre of a variable circle S which touches S1 internally and S2 externally always passes through the points:
(a) (1/2, ± √5/2)
(b) (2, ± 3/2)
(c) (1, ± 2)
(d) (0, ± √3)
9. Let the centroid of an equilateral triangle ABC be at the origin. Let one of the sides of the equilateral triangle be along the straight line x + y = 3. If R and r be the radius of circumcircle and incircle respectively of ΔABC, then (R + r) is equal to
(a) 2√2
(b) 3√2
(c) 7√2
(d) 9/√2
10. In a triangle ABC, if vector BC = 8, CA = 7, AB = 10, then the projection of the vector AB on AC is equal to:
(a) 25/4
(b) 85/14
(c) 127/20
(d) 115/16
11. Let in a Binomial distribution, consisting of 5 independent trials, probabilities of exactly 1 and 2 successes be 0.4096 and 0.2048 respectively. Then the probability of getting exactly 3 successes is equal to:
(a) 80/243
(b) 32/625
(c) 128/625
(d) 40/243
12. Let 
be two non-zero vectors perpendicular to each other and 
then the angle between the vectors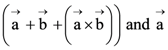 
is equal to:
(a) sin−1(1/√3)
(b) cos−1(1/√3)
(c) sin−1(1/√6)
(d) cos−1(1/√2)
13. Let a complex number be w = 1 − √3i. Let another complex number z be such that |zw| = 1 and arg (z) − arg (w) = π/2. Then the area of the triangle with vertices origin, z and w is equal to:
(a) 1/2
(b) 4
(c) 2
(d) 1/4
14. The area bounded by the curve 4y2 = x2 (4 − x) (x − 2) is equal to:
(a) 3π/2
(b) π/16
(c) π/8
(d) 3π/8
15. Define a relation R over a class of n × n real matrices A and B as “ARB if there exists a non-singular matrix P such that PAP−1 = B”. Then which of the following is true?
(a) R is reflexive, symmetric but not transitive
(b) R is symmetric, transitive but not reflexive
(c) R is an equivalence relation
(d) R is reflexive, transitive but not symmetric
16. If P and Q are two statements, then which of the following compound statement is a tautology?
(a) ((P ⇒ Q) ^ ~Q) ⇒ P
(b) ((P ⇒ Q) ^ ~ Q) ⇒ ~ P
(c) ((P ⇒ Q) ^ ~ Q)
(d) ((P ⇒ Q) ^ ~ Q) ⇒ Q
17. Consider a hyperbola H : x2 − 2y2 = 4. Let the tangent at a point P (4, √6) meet the x-axis at Q and latus rectum at R (x1, y1), x1 > 0. If F is a focus of H which is nearer to the point P, then the area of ΔQFR is equal to:
(a) √6 −1
(b) 4√6 −1
(c) 4√6
(d) 
18. Let f : R → R be a function defined as
If f is continuous at x = 0, then the value of a + b is equal to
(a) −2
(b) −2/5
(c) −3/2
(d) −3
19. Let y = y (x) be the solution of the differential equation 
0 < x < 2.1, with y(2) = 0. Then the value of 
at x = 1 is equal to:
(a)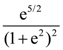 
(b)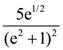 
(c) 
(d)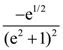 
20. Let a tangent be drawn to the ellipse 
at (3√3 cos θ, sin θ) where 
Then the value of θ such that the sum of intercepts on axes made by a tangent is minimum is equal to:
(a) π/8
(b) π/6
(c) π/3
(d) π/4
Section-B
21. Let P be a plane containing the line 
and parallel to the line 
If the point (1, −1, α) lies on the plane P, then the value of |5α| is equal to __________.
22. 
Then the value of α is equal to _________.
23. The term independent of x in the expansion of 
is equal to ________.
24. Let nCr denote the binomial coefficient of xr in the expansion of (1 + x)n. If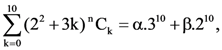 
α, β ∈ R, then α + β is equal to ________.
25. Let P (x) be a real polynomial of degree 3 which vanishes at x = − Let P(x) have local minima at x = 1, local maxima at x = −1 and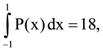 
then the sum of all the coefficients of the polynomial P (x) is equal to ____________.
26. Let the mirror image of the point (1, 3, a) with respect the plane 
be (−3, 5, 2). Then, the value of |a + b| is equal to________.
27. If f (x) and g (x) are two polynomials such that the polynomial P (x) = f (x3) + x g (x3) is divisible by x2 + x + 1, then P (1) is equal to _______.
28. Let I be an identity matrix of order 2 × 2 and 
Then the value of n ∈ N for which Pn = 5I – 8P is equal to________.
29. Let f : R → R satisfy the equation f (x + y) = f (x) . f (y) for all x, y ∈ R and f (x) ≠ 0 for any x ∈ If the function f is differentiable at x = 0 and f’ (0) = 3, then 
is equal to ________.
30. Let y = y (x) be the solution of the differential equation 
with y(1) = 0. If the area bounded by the line x = 1, x = eπ, y = 0 and y = y(x) is αe2π + b, then the value of 10(α + β) is equal to_________.
Latest Govt Job & Exam Updates: